
Why is cross product not commutative?
Answer
459.3k+ views
Hint: The cross product is defined for two vectors. For showing that it is not communicative, we have to consider two vectors
Complete step by step solution:
Let us consider two vectors
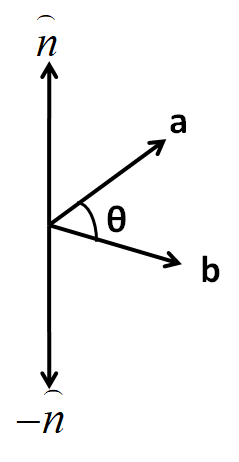
The magnitude of the cross product between the two vectors
And the direction of the cross product vector is in a direction perpendicular to the plane formed by the two vectors
If we turn the fingers of our right hand in a direction directed from the first vector
By using the above right hand thumb rule in the above figure, we get the direction of the cross product
Now, if we consider the cross product
For the direction of
From (i) and (ii) we can say that
From the above equation, we can conclude that the cross product is not communicative.
Note: Since the cross product of the two vectors has both the direction and the magnitude, it is also known as the vector product. We must note that only the direction of the vectors
Complete step by step solution:
Let us consider two vectors

The magnitude of the cross product between the two vectors
And the direction of the cross product vector is in a direction perpendicular to the plane formed by the two vectors
If we turn the fingers of our right hand in a direction directed from the first vector
By using the above right hand thumb rule in the above figure, we get the direction of the cross product
Now, if we consider the cross product
For the direction of
From (i) and (ii) we can say that
From the above equation, we can conclude that the cross product is not communicative.
Note: Since the cross product of the two vectors has both the direction and the magnitude, it is also known as the vector product. We must note that only the direction of the vectors
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




