Question

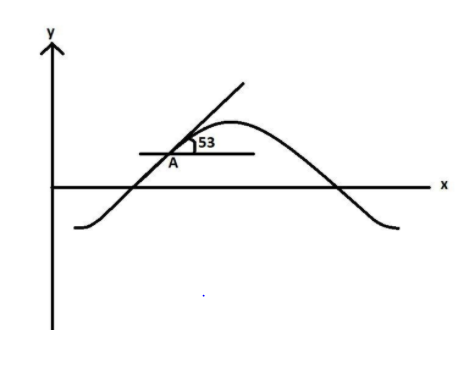
Curve 'yx' at an instant for a wave travelling along x-axis on a string is shown. Slope at the point A on the curve, as shown, is 53$^{\circ }$
 

(A). Transverse velocity of the particle at point A is positive if the wave is travelling along the positive x−axis.
(B). Transverse velocity of the particle at point A is positive if the wave is travelling along negative x−axis of the particle at point A
(C). Magnitude of the transverse velocity of the particle at point A is greater than wave speed.
(D). Magnitude of transverse velocity of the particle at point A is lesser than wave speed.

Answer 1

Hint: Analyse the graph at first, this is basically a time and velocity graph. We know that the velocity of a particle is represented as a negative gradient of time and wave velocity. Now take each and every option and equate the options with the equation we will get to know which option is correct and which is not.

Complete step-by-step answer:
The velocity of the particle is represented as,
${{V}_{p}}=-\dfrac{dy}{dx}{{V}_{w}}$ , here ${{V}_{w}}$ is the velocity of the wave, and $\dfrac{dy}{dx}$ is the time.
In the first option that is option A, it says that Transverse velocity of the particle at point A is positive if the wave is traveling along the positive x−axis,
And from the above equation of velocity of the particle, we figure out that when the velocity of the wave is positive then the velocity of the particle is negative, hence option A is wrong.
Now considering the second option that says that the transverse velocity of the particle at point A is positive if the wave is traveling along the negative x−axis of the particle at point A.
When the wave is traveling along the negative axis from the above equation we can say that the velocity of the particle is positive.

Hence option B is correct.
Now we are considering the third option that is, the magnitude of the transverse velocity of the particle at point A is greater than the wave speed.
So we know that time is represented in the equation by $-\dfrac{dy}{dx}$ , and we know that slope at point A on the curve is 53$^{\circ }$ ,

That equals 5/3.
Now we know that,
5/3 is greater than 1.
Hence the value of the velocity of the particle is greater than the velocity of the wave,
Hence, option C is also the correct option.
And now considering the last option D, which states Magnitude of the transverse velocity of the particle at point A is lesser than the wave speed.
While solving option c we already figured out that at a point A of the slope velocity of the particle is more than than the velocity of the wave.
So option D is wrong.
Therefore, Option B and Option C is the correct option.

Note: When evaluating option C, we get that the time variable is greater than 1, so the velocity of the particle must be greater than the velocity of the wave as the velocity of the particle is the product of time and wave velocity.