
Define an identity function and draw its graph, also find its domain and range.
Answer
426k+ views
Hint:We are given a question asking us to define an identity function and then graph it and further, find the function’s domain and range. An identity function is one that has each of its elements in the domain and has an image of itself in the range. The function for the same can be defined as,
Complete step by step answer:
According to the given question, we are asked to define an identity function and draw the graph of the respective function and then we have to mention the function’s domain and range.
An identity function is one that has each of its elements in the domain and has an image of itself as the range.
The function can be defined as,
That is,
So, we have the function as,
We will draw the graph of this function and for that, we will plot some points and we have,
That is, for any value of ‘x’, the function has same value.
We get the graph as,
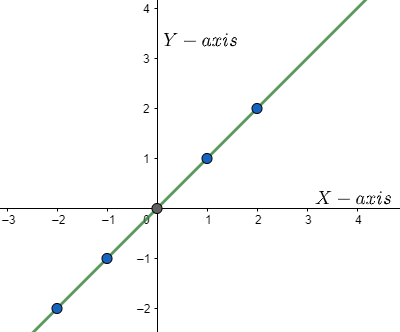
We can see that the graph is a straight line passing through the origin.
And it is defined from the set of real numbers. So, we have the domain and the range as the set of all real numbers, that is,
Domain of the function = R
Range of the function = R
Note: The identity function should be confused with the function having 1 in the range. Also, to graph any function, always find the coordinates first and not do it directly, so as to reduce the risk of getting the graph wrong. The domain refers to the values of the ‘x’ and range refers to the values of
Complete step by step answer:
According to the given question, we are asked to define an identity function and draw the graph of the respective function and then we have to mention the function’s domain and range.
An identity function is one that has each of its elements in the domain and has an image of itself as the range.
The function can be defined as,
That is,
So, we have the function as,
We will draw the graph of this function and for that, we will plot some points and we have,
| -2 | -1 | 0 | 1 | 2 | |
| -2 | -1 | 0 | 1 | 2 |
That is, for any value of ‘x’, the function has same value.
We get the graph as,

We can see that the graph is a straight line passing through the origin.
And it is defined from the set of real numbers. So, we have the domain and the range as the set of all real numbers, that is,
Domain of the function = R
Range of the function = R
Note: The identity function should be confused with the function having 1 in the range. Also, to graph any function, always find the coordinates first and not do it directly, so as to reduce the risk of getting the graph wrong. The domain refers to the values of the ‘x’ and range refers to the values of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




