
Define injective function. Give an example of injective function.
Answer
485.1k+ views
Hint: We solve this problem by defining the injective function and then we take some examples.
The injective function is also called as one – one function which is defined as “for every element in the codomain there is the image of exactly one element in the domain”. The graphical representation of a function

Here, X is the domain and the set Y is called the codomain.
By using this definition we take some examples of injective functions.
Complete step by step answer:
We are asked to define the injective function.
The injective function is defined as a function in which for every element in the codomain there is an image of exactly one in the domain.
Let us assume that a function mapping as
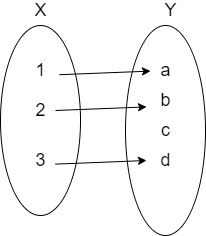
Here, we can see that for every element in the domain X there is exactly one image in the codomain Y.
So, we can say that this function is an injective function.
There is other definition of injective in mathematical form as follows:
“A function of domain X is said to an injective function if for any two elements
Symbolically we can say that
Now, let us take one example as follows
(1) The function
Let us check whether this function is injective or not.
Let us assume two values
Now by using the function
We know that if a function is defined as
By using this definition we can conclude that the function
Let us take some more examples of injective functions as follows:
(2) The function
(3) The function
Note: We come across some situations where we are asked to check whether a given function is injective or not. Then we use the mathematical definition in the case that is
But students may do from the direct definition that is “for every element in the codomain there is the image of exactly one element in the domain”
Here we need to check for each and every element in the domain.
If the function is mapped as
So, we need to use the mathematical definition for solving the problem easily.
The injective function is also called as one – one function which is defined as “for every element in the codomain there is the image of exactly one element in the domain”. The graphical representation of a function

Here, X is the domain and the set Y is called the codomain.
By using this definition we take some examples of injective functions.
Complete step by step answer:
We are asked to define the injective function.
The injective function is defined as a function in which for every element in the codomain there is an image of exactly one in the domain.
Let us assume that a function mapping as

Here, we can see that for every element in the domain X there is exactly one image in the codomain Y.
So, we can say that this function is an injective function.
There is other definition of injective in mathematical form as follows:
“A function of domain X is said to an injective function if for any two elements
Symbolically we can say that
Now, let us take one example as follows
(1) The function
Let us check whether this function is injective or not.
Let us assume two values
Now by using the function
We know that if a function is defined as
By using this definition we can conclude that the function
Let us take some more examples of injective functions as follows:
(2) The function
(3) The function
Note: We come across some situations where we are asked to check whether a given function is injective or not. Then we use the mathematical definition in the case that is
But students may do from the direct definition that is “for every element in the codomain there is the image of exactly one element in the domain”
Here we need to check for each and every element in the domain.
If the function is mapped as
So, we need to use the mathematical definition for solving the problem easily.
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




