
What is the definition of exponential decay?
Answer
443.7k+ views
Hint: A quantity is subjected to exponential decay if it decreases at a rate proportional to its current value. Exponential decay is the decrease in a quantity N according to the law
Complete step-by-step answer:
Let us see the definition of exponential decay. We can say that a quantity is subjected to exponential decay if it decreases at a rate proportional to its current value. The decay law calculates the number of undecayed nuclei in a given radioactive substance.
Let us see the formula for Half-Life in Exponential Decay. Half-life is the length of time it takes an exponentially decaying quantity to decrease to half its original amount. The formula for Half-Life in Exponential Decay is given as
where
We can also write the decay formula as
where
We mainly use the below formula for exponential decay.
Exponential decay is described by the first-order ordinary differential equation.
Let us rearrange this equation.
Let us integrate both the sides.
We know that
Let us take exponents on both the sides.
We know that
Let us denote
where
We can also relate the parameters
When comparing the above three formulas, we will get
Let us take the natural logarithms on both sides.
We know that
Let us cancel t from all the terms.
Let us consider
We know that
We know that
Let us cancel the negative sign from both the sides.
Let us take
Now, let us consider
Similarly, when we solve the LHS, we will get
Let us take
From (i) and (ii), we can write
Let us consider
We know that
Let us take
Decay constant (
The graph of exponential decay is shown below for the function
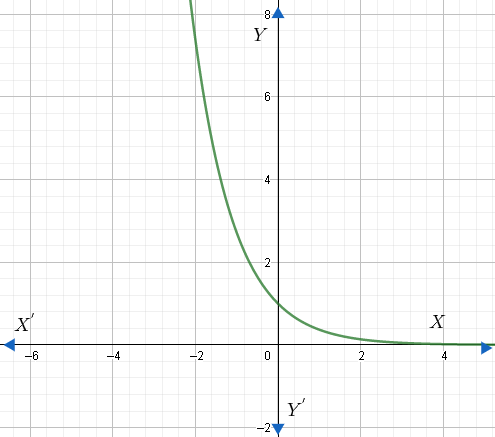
Therefore, exponential decay is the decrease in a quantity N according to the law
Note: Students may get confused with exponential growth and exponential decay. xponential growth occurs when the growth rate of the value of a mathematical function is proportional to the function’s current value, resulting in its growth with time being an exponential function. The formula for exponential growth is
Complete step-by-step answer:
Let us see the definition of exponential decay. We can say that a quantity is subjected to exponential decay if it decreases at a rate proportional to its current value. The decay law calculates the number of undecayed nuclei in a given radioactive substance.
Let us see the formula for Half-Life in Exponential Decay. Half-life is the length of time it takes an exponentially decaying quantity to decrease to half its original amount. The formula for Half-Life in Exponential Decay is given as
where
We can also write the decay formula as
where
We mainly use the below formula for exponential decay.
Exponential decay is described by the first-order ordinary differential equation.
Let us rearrange this equation.
Let us integrate both the sides.
We know that
Let us take exponents on both the sides.
We know that
Let us denote
where
We can also relate the parameters
When comparing the above three formulas, we will get
Let us take the natural logarithms on both sides.
We know that
Let us cancel t from all the terms.
Let us consider
We know that
We know that
Let us cancel the negative sign from both the sides.
Let us take
Now, let us consider
Similarly, when we solve the LHS, we will get
Let us take
From (i) and (ii), we can write
Let us consider
We know that
Let us take
Decay constant (
The graph of exponential decay is shown below for the function

Therefore, exponential decay is the decrease in a quantity N according to the law
Note: Students may get confused with exponential growth and exponential decay. xponential growth occurs when the growth rate of the value of a mathematical function is proportional to the function’s current value, resulting in its growth with time being an exponential function. The formula for exponential growth is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




