
Derive an expression for drift velocity of free electrons in a conductor.
Answer
433k+ views
11 likes
Hint: Consider electrons as infinitesimal free particles in the conductor experiencing force due to an electric field. We can calculate the force on each electron, and find the acceleration. Drift velocity is the average velocity of all the electrons in a given space.
Formula used:
Force on a charged particle in an electric field is given by,
Where,
The general kinematics equation,
Where,
and
Complete step by step answer:
Let’s assume we have an electric field E in the conductor.
For now, we are going to consider only one electron, and calculate the acceleration of the electron due to this electric field.
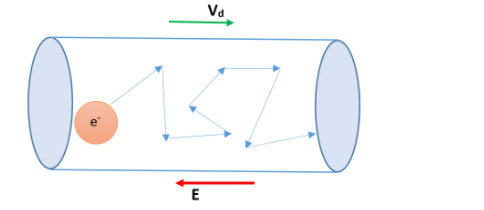
As the force due to the electric field is much more than the electron-electron interaction, we are not going to consider that.
Let’s consider the charge of an electron to be (-e).
Hence, the force on the electron is equal to
We can find the acceleration of the electron by,
Where m is the mass of the electron.
Now, let us introduce a quantity called the relaxation time. It is the average time between two consecutive collisions.
Let’s assume there are ‘n’ number of electrons in the conductor at any given time.
The relaxation time is given by,
Where,
We can say that the drift velocity is the average velocity of all the electrons.
So, we can write,
We can use the general kinematics equation,
Where,
and
Using equation (2) we get,
The expression of drift velocity is,
Note:
Students should be clear with the approach. If you know the basic equations, you can easily derive to the above expression. We all know that the vibration of the atoms increases as we heat a conductor. So, the time duration between two collisions decreases. As a result, the relaxation time decreases. The drift velocity is directly proportional to the relaxation time. So, drift velocity will decrease with the increase in temperature.
Formula used:
Force on a charged particle in an electric field is given by,
Where,
The general kinematics equation,
Where,
and
Complete step by step answer:
Let’s assume we have an electric field E in the conductor.
For now, we are going to consider only one electron, and calculate the acceleration of the electron due to this electric field.

As the force due to the electric field is much more than the electron-electron interaction, we are not going to consider that.
Let’s consider the charge of an electron to be (-e).
Hence, the force on the electron is equal to
We can find the acceleration of the electron by,
Where m is the mass of the electron.
Now, let us introduce a quantity called the relaxation time. It is the average time between two consecutive collisions.
Let’s assume there are ‘n’ number of electrons in the conductor at any given time.
The relaxation time is given by,
Where,
We can say that the drift velocity is the average velocity of all the electrons.
So, we can write,
We can use the general kinematics equation,
Where,
and
Using equation (2) we get,
The expression of drift velocity is,
Note:
Students should be clear with the approach. If you know the basic equations, you can easily derive to the above expression. We all know that the vibration of the atoms increases as we heat a conductor. So, the time duration between two collisions decreases. As a result, the relaxation time decreases. The drift velocity is directly proportional to the relaxation time. So, drift velocity will decrease with the increase in temperature.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




