
Derive an expression for mechanical force per unit area of surface charge density.
Answer
475.8k+ views
Hint: The quantity of charge contained per unit area is called surface charge density. All charged conductors will experience some mechanical force. Elements having a surface charge density will always experience a mechanical force outwards. Here we have to derive an expression for the mechanical force.
Complete step by step answer:
Small elements of the conductor will experience a mechanical force normally outwards. Let us consider the small element
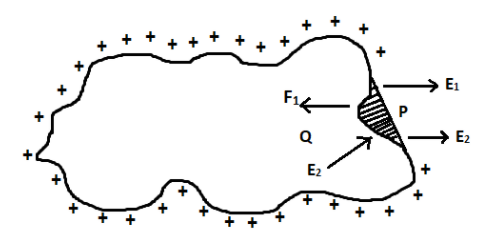
Let
Then we can write,
Let us consider a point
At this point
where
The electrical intensity is directed normally outwards.
The electrical intensity
Hence the total electrical intensity at the point
Substituting this value in equation
Let us now consider the point
i.e.
Substituting
We get
This can be written as,
From this, we can write
We know that the electrical intensity due to the rest of the charges in the conductor is
The repulsive force experienced by the rest of the conductor due to the charge
We know that
and
Substituting these values in the equation for force,
From this equation, we can write
The force per unit area can be written as,
Thus the above equation will become,
This is the mechanical force per unit area of a conductor due to a surface charge density.
Note: The S.I unit for the mechanical force per unit area is given by
Complete step by step answer:
Small elements of the conductor will experience a mechanical force normally outwards. Let us consider the small element

Let
Then we can write,
Let us consider a point
At this point
where
The electrical intensity is directed normally outwards.
The electrical intensity
Hence the total electrical intensity at the point
Substituting this value in equation
Let us now consider the point
i.e.
Substituting
We get
This can be written as,
From this, we can write
We know that the electrical intensity due to the rest of the charges in the conductor is
The repulsive force experienced by the rest of the conductor due to the charge
We know that
and
Substituting these values in the equation for force,
From this equation, we can write
The force per unit area can be written as,
Thus the above equation will become,
This is the mechanical force per unit area of a conductor due to a surface charge density.
Note: The S.I unit for the mechanical force per unit area is given by
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




