
Derive Einstein’s photoelectric equation
Answer
486.9k+ views
2 likes
Hint:Photoelectric effect is the phenomenon in which when the light strikes the metal plate and then it releases the electrons. When a light strikes the surface then some of the light is absorbed, some gets reflected, the absorbed light is responsible for the electron emission.
Formula used:The formula of the kinetic energy is given by,
Where the mass of the object is equal to m and the velocity of the object is equal to v.
The formula of the incident photon is given by,
Where the Planck’s constant is h and the frequency is equal to v.
Complete step by step solution:
It is asked in the problem to derive Einstein's photoelectric equation.
When a beam of light strikes the surface of the metal then the electrons will release from the surface of the electron. The energy of the photon which is required to eject the electrons is equal to threshold energy for the removal of the electron and also the kinetic energy of the electron with which the electron leaves the metal surface. The electron must have some kinetic energy when it leaves the surface of the metal if the electrons don’t have the required kinetic energy then there is no use of the ejection of electron and therefore the energy of the electron must be given such that it has threshold energy for the ejection of electron and also it has the energy that the electrons leaves the metal surface with maximum kinetic energy.
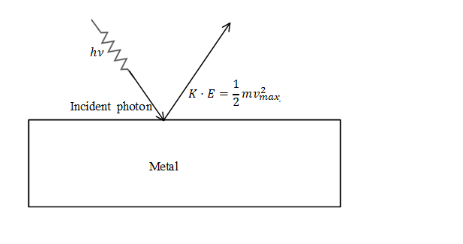
The energy of the photons is equal to,
Energy of the photon= Energy required to eject the electrons Maximum kinetic energy of electron.
The energy of the photon is equal to,
Where Planck’s constant is h and frequency is v.
The energy required for the ejection of the electrons is equal to,
Where Planck’s constant is h and the minimum threshold frequency is
The equation of the Einstein’s photoelectric equation is equal to,
Note:The students are advised to understand and remember the formula of the kinetic energy and also the formula of the energy of the photon as these formulas are very important in solving these types of problems.
Formula used:The formula of the kinetic energy is given by,
Where the mass of the object is equal to m and the velocity of the object is equal to v.
The formula of the incident photon is given by,
Where the Planck’s constant is h and the frequency is equal to v.
Complete step by step solution:
It is asked in the problem to derive Einstein's photoelectric equation.
When a beam of light strikes the surface of the metal then the electrons will release from the surface of the electron. The energy of the photon which is required to eject the electrons is equal to threshold energy for the removal of the electron and also the kinetic energy of the electron with which the electron leaves the metal surface. The electron must have some kinetic energy when it leaves the surface of the metal if the electrons don’t have the required kinetic energy then there is no use of the ejection of electron and therefore the energy of the electron must be given such that it has threshold energy for the ejection of electron and also it has the energy that the electrons leaves the metal surface with maximum kinetic energy.

The energy of the photons is equal to,
Energy of the photon= Energy required to eject the electrons Maximum kinetic energy of electron.
The energy of the photon is equal to,
Where Planck’s constant is h and frequency is v.
The energy required for the ejection of the electrons is equal to,
Where Planck’s constant is h and the minimum threshold frequency is
The equation of the Einstein’s photoelectric equation is equal to,
Note:The students are advised to understand and remember the formula of the kinetic energy and also the formula of the energy of the photon as these formulas are very important in solving these types of problems.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




