
Derive the expression for motional EMF induced in a conductor moving in a uniform magnetic field.
Answer
436.6k+ views
6 likes
Hint: The magnetic flux enclosed in area A is given by
If the length of the conductor is l and displacement be x then the area A is given by
Hence,
Complete step by step solution:
1) Consider a straight conducting wire placed in an uniform magnetic field. The length of the wire is AB= l.
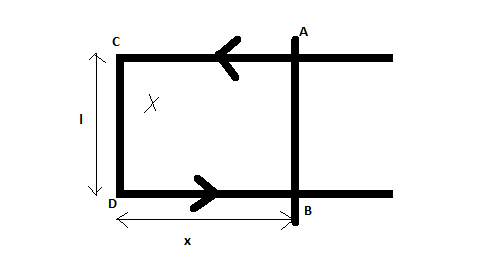
2) Now it is shifted x distance to another point CD.
3) Hence the flux associated with the loop ABCD is given by
4) As the displacement x changes with time, then there is a rate of change of induced flux. According to Faraday’s law, this rate of change of flux will induce an emf in the conductor. The rate of change of flux is hence given by
This induced emf is called the motional emf.
Note: The negative sign given here indicates that this emf opposes the cause of its generation. Faraday’s law of electromagnetic induction gives the concept of motional emf which is different from the emf of the cell and opposes it.
If the length of the conductor is l and displacement be x then the area A is given by
Hence,
Complete step by step solution:
1) Consider a straight conducting wire placed in an uniform magnetic field. The length of the wire is AB= l.

2) Now it is shifted x distance to another point CD.
3) Hence the flux associated with the loop ABCD is given by
4) As the displacement x changes with time, then there is a rate of change of induced flux. According to Faraday’s law, this rate of change of flux will induce an emf in the conductor. The rate of change of flux is hence given by
This induced emf is called the motional emf.
Note: The negative sign given here indicates that this emf opposes the cause of its generation. Faraday’s law of electromagnetic induction gives the concept of motional emf which is different from the emf of the cell and opposes it.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




