
Derive the expression for the potential energy of a system of two charges in the absence of the external electric field.
Answer
506.1k+ views
5 likes
Hint: The potential energy of this system of charge is equal to total work done ,i.e., To move charge q
Complete step-by-step answer:
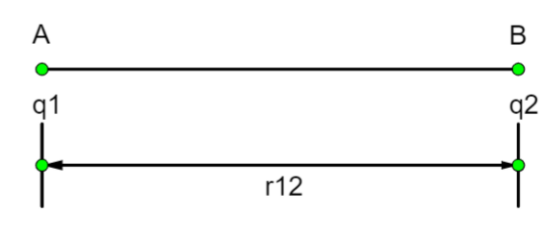
The work done to move q from infinity to A is,
(
The work done to move q
Here,
The potential energy of this system of charge is equal to total work done to bring the charges from infinity to A or B.
Note: Generally students can go wrong in considering the work done to move charge q
Complete step-by-step answer:

The work done to move q from infinity to A is,
(
The work done to move q
Here,
The potential energy of this system of charge is equal to total work done to bring the charges from infinity to A or B.
Note: Generally students can go wrong in considering the work done to move charge q
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




