
Derive the formula for the force acting between two parallel current carrying conductors.
Answer
479.1k+ views
Hint: Before we proceed to derive the expression for force acting between two current carrying parallel conductors, we should be familiar with the expression for the Biot-Savart’s law which gives the expression for magnetic field at a point due to a current carrying conductor, as follows –
where I = current in the circuit,
Complete step by step solution:
Consider two conductors A and B of infinite length, with currents
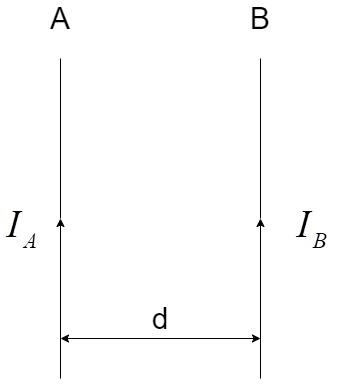
The current
Whenever a current carrying conductor is placed in an external magnetic field, there is a force acting on it and the force experienced by the conductor is equal to the product of magnetic field, current and the length of the conductor.
The conductor B experiences force due to the magnetic field produced by the conductor A, which is given by
Therefore, the force acting on the conductor B will be equal to –
Substituting the value
Therefore, the force per unit length between two parallel conductors is given by the expression:
The direction of the force can be calculated by using the Fleming’s Left Hand rule where:
i) Index finger represents the direction of the magnetic field, B
ii) Middle finger represents the direction of the current, I
iii) Thumb represents the direction of the force, F
The direction of the force is represented in the diagram below:
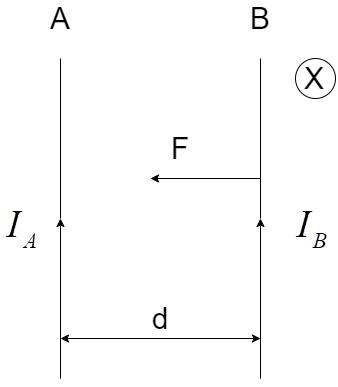
Here, the direction of
Note: The standard definition of the SI unit of current, ampere is defined by the help of the derived expression as follows:
If
Thus, One ampere is defined as the steady current, which when maintained between two infinitely long conductors with unit separation, would produce a force per unit length equal to
where I = current in the circuit,
Complete step by step solution:
Consider two conductors A and B of infinite length, with currents

The current
Whenever a current carrying conductor is placed in an external magnetic field, there is a force acting on it and the force experienced by the conductor is equal to the product of magnetic field, current and the length of the conductor.
The conductor B experiences force due to the magnetic field produced by the conductor A, which is given by
Therefore, the force acting on the conductor B will be equal to –
Substituting the value
Therefore, the force per unit length between two parallel conductors is given by the expression:
The direction of the force can be calculated by using the Fleming’s Left Hand rule where:
i) Index finger represents the direction of the magnetic field, B
ii) Middle finger represents the direction of the current, I
iii) Thumb represents the direction of the force, F
The direction of the force is represented in the diagram below:

Here, the direction of
Note: The standard definition of the SI unit of current, ampere is defined by the help of the derived expression as follows:
If
Thus, One ampere is defined as the steady current, which when maintained between two infinitely long conductors with unit separation, would produce a force per unit length equal to
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




