
Derive the formula for the volume of the frustum of a cone.
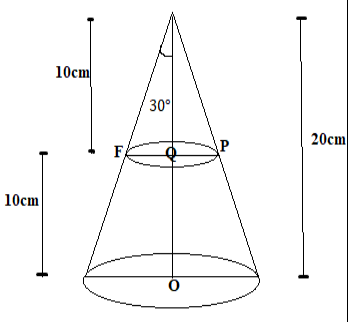

Answer
477.9k+ views
Hint: A cone is a three-dimensional geometric shape that tapers smoothly from a flat base to a point called the apex or vertex and its volume is given by, $\dfrac{1}{3}\pi {r^2}h$
While, a frustum is the portion of a solid that lies between one or two parallel planes cutting.
A right frustum is a parallel truncation of a right pyramid or right cone. So to solve this particular question we just have to subtract the volume of a smaller portion of the cone from the whole volume of the cone.
Complete step-by-step answer:
In order to solve the question, let us mark all the edges and points of cone for our simplicity as shown below,
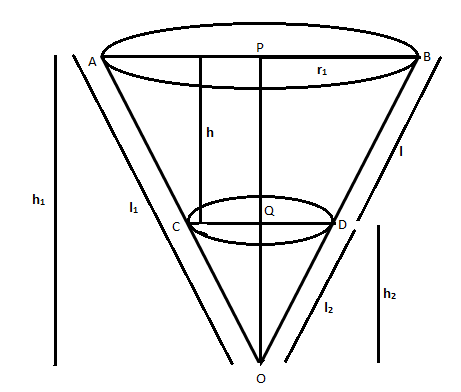
There are two cones \[OCD\]&\[OAB\],
We are given
Height of frustum \[ = h\]
Slant height of frustum \[ = l\]
Radius \[PB = {\text{ }}{r_1}\]
Radius \[QB = {\text{ }}{r_2}\]
We need to find
Curved Surface Area and Total surface Area
Here,
We need to write \[\;{h_1},{\text{ }}{l_1},{\text{ }}{h_2},{\text{ }}{l_2}\] in terms of \[h\] and \[l\]
Volume of frustum = Volume of cone OAB - Volume of cone OCD \[ = \dfrac{1}{3}\pi {r_1}^2{h_1} - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}....(1)\]\[\Delta {\text{OPB & }}\Delta {\text{OQD}}\]
\[\angle BOP = \angle DOQ\] (Common)
\[\angle OPB = \angle OQD\] (Both are \[{90^o}\] as heights are perpendicular)
\[{\text{So, }}\Delta {\text{OPB ~ }}\Delta {\text{OQD}}\]
\[\therefore {\text{ }}\dfrac{{{\text{PB}}}}{{{\text{QD}}}}{\text{ = }}\dfrac{{{\text{OB}}}}{{{\text{OD}}}}\]
Since, Corresponding sides of similar triangles are proportional
\[\therefore {\text{ }}\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{{{h_1}}}{{{h_2}}}\]
Putting, \[{h_1} = h + {h_2}\]
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{{h + {h_2}}}{{{h_2}}}\]
We split the RHS we get,
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{h}{{{h_2}}} + \dfrac{{{h_2}}}{{{h_2}}}\]
On cancel the term we get,
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{h}{{{h_2}}} + 1\]
Taking 1 on RHS we get,
\[\dfrac{{{r_1}}}{{{r_2}}} - 1 = \dfrac{h}{{{h_2}}}\]
Let us take LCM we get,
\[\dfrac{{{r_1} - {r_2}}}{{{r_2}}} = \dfrac{h}{{{h_2}}}\]
We can take a cross multiplication we get,
\[{h_2}\left( {\dfrac{{{r_1} - {r_2}}}{{{r_2}}}} \right) = h\]
Again we do cross multiplication we get,
\[{h_2} = h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)....(2)\]
From\[\left( 1 \right)\], Volume of the frustum\[ = \dfrac{1}{3}\pi {r_1}^2{h_1} - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}\]
putting, \[{h_1} = h + {h_2}\]
Volume of the frustum \[{\text{ }} = \dfrac{1}{3}\pi {r_1}^2\left( {h + {h_2}} \right) - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}\]
From \[\left( 2 \right)\]: Putting \[{h_2} = h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
\[ = \dfrac{1}{3}\pi {r_1}^2\left( {h + h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
Taking h as common on the terms we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {1 + \left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
Let us take the LCM of the first term we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {\dfrac{{{r_1} - {r_2} + {r_2}}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
On some simplification in the numerator term we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {\dfrac{{{r_1}}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
On multiplying \[r\] terms we get,
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{{r_1}^3}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi h\left( {\dfrac{{{r_2}^3}}{{{r_1} - {r_2}}}} \right)\]
Let us take the common terms we get,
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{{r_1}^3 - {r_2}^3}}{{{r_1} - {r_2}}}} \right)\]
Using the formula, \[{{\text{a}}^{\text{3}}}{\text{ - }}{{\text{b}}^{\text{3}}}{\text{ = (a - b)(}}{{\text{a}}^{\text{2}}}{\text{ + }}{{\text{b}}^{\text{2}}}{\text{ + ab)}}\] in the above term becomes
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{({r_1} - {r_2})({r_1}^2 + {r_2}^2 + {r_1}{r_2})}}{{{r_1} - {r_2}}}} \right)\]
On cancel the same terms we get,
\[ = \dfrac{1}{3}\pi h({r_1}^2 + {r_2}^2 + {r_1}{r_2})\]
$\therefore $ Volume of frustum=\[ = \dfrac{1}{3}\pi h({r_1}^2 + {r_2}^2 + {r_1}{r_2})\]
Hence we proved the formula for the volume of the frustum of a cone.
Note: In geometry, a frustum is the portion of a solid (normally a cone or pyramid) that lies between one or two parallel planes cutting it.
Here, we have to find the volume of the cone. Volume is the quantity of three-dimensional space enclosed by a closed surface of the frustum of a cone.
Therefore, basic knowledge about geometric shapes must be known by any student. Like in this problem, students shouldn’t be confused with whose volume is to be determined.
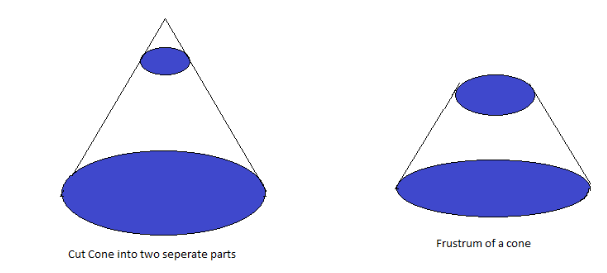
While, a frustum is the portion of a solid that lies between one or two parallel planes cutting.
A right frustum is a parallel truncation of a right pyramid or right cone. So to solve this particular question we just have to subtract the volume of a smaller portion of the cone from the whole volume of the cone.
Complete step-by-step answer:
In order to solve the question, let us mark all the edges and points of cone for our simplicity as shown below,

There are two cones \[OCD\]&\[OAB\],
We are given
Height of frustum \[ = h\]
Slant height of frustum \[ = l\]
Radius \[PB = {\text{ }}{r_1}\]
Radius \[QB = {\text{ }}{r_2}\]
We need to find
Curved Surface Area and Total surface Area
Here,
We need to write \[\;{h_1},{\text{ }}{l_1},{\text{ }}{h_2},{\text{ }}{l_2}\] in terms of \[h\] and \[l\]
Volume of frustum = Volume of cone OAB - Volume of cone OCD \[ = \dfrac{1}{3}\pi {r_1}^2{h_1} - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}....(1)\]\[\Delta {\text{OPB & }}\Delta {\text{OQD}}\]
\[\angle BOP = \angle DOQ\] (Common)
\[\angle OPB = \angle OQD\] (Both are \[{90^o}\] as heights are perpendicular)
\[{\text{So, }}\Delta {\text{OPB ~ }}\Delta {\text{OQD}}\]
\[\therefore {\text{ }}\dfrac{{{\text{PB}}}}{{{\text{QD}}}}{\text{ = }}\dfrac{{{\text{OB}}}}{{{\text{OD}}}}\]
Since, Corresponding sides of similar triangles are proportional
\[\therefore {\text{ }}\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{{{h_1}}}{{{h_2}}}\]
Putting, \[{h_1} = h + {h_2}\]
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{{h + {h_2}}}{{{h_2}}}\]
We split the RHS we get,
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{h}{{{h_2}}} + \dfrac{{{h_2}}}{{{h_2}}}\]
On cancel the term we get,
\[\dfrac{{{r_1}}}{{{r_2}}} = \dfrac{h}{{{h_2}}} + 1\]
Taking 1 on RHS we get,
\[\dfrac{{{r_1}}}{{{r_2}}} - 1 = \dfrac{h}{{{h_2}}}\]
Let us take LCM we get,
\[\dfrac{{{r_1} - {r_2}}}{{{r_2}}} = \dfrac{h}{{{h_2}}}\]
We can take a cross multiplication we get,
\[{h_2}\left( {\dfrac{{{r_1} - {r_2}}}{{{r_2}}}} \right) = h\]
Again we do cross multiplication we get,
\[{h_2} = h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)....(2)\]
From\[\left( 1 \right)\], Volume of the frustum\[ = \dfrac{1}{3}\pi {r_1}^2{h_1} - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}\]
putting, \[{h_1} = h + {h_2}\]
Volume of the frustum \[{\text{ }} = \dfrac{1}{3}\pi {r_1}^2\left( {h + {h_2}} \right) - \dfrac{1}{3}\pi {r_2}^2{h_{^2}}\]
From \[\left( 2 \right)\]: Putting \[{h_2} = h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
\[ = \dfrac{1}{3}\pi {r_1}^2\left( {h + h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
Taking h as common on the terms we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {1 + \left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
Let us take the LCM of the first term we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {\dfrac{{{r_1} - {r_2} + {r_2}}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
On some simplification in the numerator term we get,
\[ = \dfrac{1}{3}\pi {r_1}^2h\left( {\dfrac{{{r_1}}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi {r_2}^2h\left( {\dfrac{{{r_2}}}{{{r_1} - {r_2}}}} \right)\]
On multiplying \[r\] terms we get,
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{{r_1}^3}}{{{r_1} - {r_2}}}} \right) - \dfrac{1}{3}\pi h\left( {\dfrac{{{r_2}^3}}{{{r_1} - {r_2}}}} \right)\]
Let us take the common terms we get,
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{{r_1}^3 - {r_2}^3}}{{{r_1} - {r_2}}}} \right)\]
Using the formula, \[{{\text{a}}^{\text{3}}}{\text{ - }}{{\text{b}}^{\text{3}}}{\text{ = (a - b)(}}{{\text{a}}^{\text{2}}}{\text{ + }}{{\text{b}}^{\text{2}}}{\text{ + ab)}}\] in the above term becomes
\[ = \dfrac{1}{3}\pi h\left( {\dfrac{{({r_1} - {r_2})({r_1}^2 + {r_2}^2 + {r_1}{r_2})}}{{{r_1} - {r_2}}}} \right)\]
On cancel the same terms we get,
\[ = \dfrac{1}{3}\pi h({r_1}^2 + {r_2}^2 + {r_1}{r_2})\]
$\therefore $ Volume of frustum=\[ = \dfrac{1}{3}\pi h({r_1}^2 + {r_2}^2 + {r_1}{r_2})\]
Hence we proved the formula for the volume of the frustum of a cone.
Note: In geometry, a frustum is the portion of a solid (normally a cone or pyramid) that lies between one or two parallel planes cutting it.
Here, we have to find the volume of the cone. Volume is the quantity of three-dimensional space enclosed by a closed surface of the frustum of a cone.
Therefore, basic knowledge about geometric shapes must be known by any student. Like in this problem, students shouldn’t be confused with whose volume is to be determined.

Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What constitutes the central nervous system How are class 10 biology CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE

Explain the Treaty of Vienna of 1815 class 10 social science CBSE




