
Describe the biprism experiment to find the wavelength of the monochromatic light. Draw the necessary ray diagram.
Answer
483.9k+ views
Hint : In the biprism experiment, two coherent sources of light that are required for the interference pattern are produced with the help of two thin prisms. To find the wavelength of light we can measure the fringe width of the interference pattern that is formed from the interference of these two coherent sources.
Formula used: In this solution, we will use the following formula
Complete step by step answer
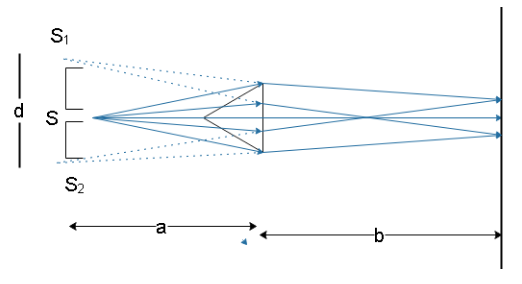
When a monochromatic light source
From the properties of interference of light waves, we know that the fringe width is
As we can see in the figure, the distance between the screen and the light source is
If the angle of the prism is represented by
Substituting the value of
Solving for the wavelength of light, we can write
In the above equation, we know the angle of the prism
Note
The biprism experiment is very similar to young’s modulus where a single source of light is split using two different slips whereas, in a biprism experiment, a biprism is used to form two apparent coherent sources. The two sources of light that interfere must be coherent with each other an interference pattern will not be formed.
Formula used: In this solution, we will use the following formula
Complete step by step answer

When a monochromatic light source
From the properties of interference of light waves, we know that the fringe width is
As we can see in the figure, the distance between the screen and the light source is
If the angle of the prism is represented by
Substituting the value of
Solving for the wavelength of light, we can write
In the above equation, we know the angle of the prism
Note
The biprism experiment is very similar to young’s modulus where a single source of light is split using two different slips whereas, in a biprism experiment, a biprism is used to form two apparent coherent sources. The two sources of light that interfere must be coherent with each other an interference pattern will not be formed.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




