
Determine the angle of minimum deviation for a given prism by plotting a graph between the angle of incidence and angle of deviation.
Answer
439.8k+ views
Hint: The refractive index can be found by taking the ratio of the sine of half of the sum of the angle of prism and angle of minimum deviation to the sine of the half of the angle of prism. Using this find the procedure to find the angle of minimum deviation and plot the graph.
Complete step by step answer:
Goal: to calculate the minimum deviation angle for a prism by plotting a graph between the angle of incidence and angle of deviation.
Theory: Refraction happens if a light ray travels from one medium to another which is resulting in the deviation of the emergent ray from that of the incident ray. The refractive index of the material used in the prism can be shown as,
Where
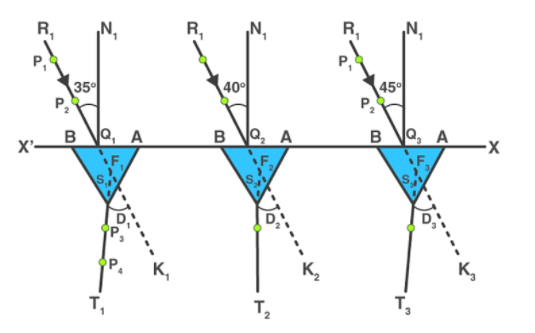
Procedure: keep the white sheet of paper on a drawing board and place it with the help of drawing pins.
Observation: the angle of prism
The graph can be plotted like this,
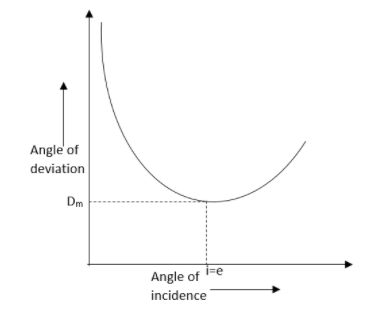
Result: the angle of minimum deviation is found. The graph has been plotted.
Note: This graph represents that the angle of incidence increases, the angle of deviation decreases first to attain the minimum value of
Complete step by step answer:
Goal: to calculate the minimum deviation angle for a prism by plotting a graph between the angle of incidence and angle of deviation.
Theory: Refraction happens if a light ray travels from one medium to another which is resulting in the deviation of the emergent ray from that of the incident ray. The refractive index of the material used in the prism can be shown as,
Where

Procedure: keep the white sheet of paper on a drawing board and place it with the help of drawing pins.
Observation: the angle of prism
| Serial no. | Angle of incidence | Angle of deviation |
| 1 | ||
| 2 | ||
| 3 | ||
| 4 | ||
| 5 | ||
| 6 |
The graph can be plotted like this,

Result: the angle of minimum deviation is found. The graph has been plotted.
Note: This graph represents that the angle of incidence increases, the angle of deviation decreases first to attain the minimum value of
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




