
Determine the force acting between two parallel current carrying conductor wires. Write a theoretical definition of ampere on this basis.
Answer
480.6k+ views
Hint: First of all, we will find out the magnetic field produced by the current in the first wire and then we will find the force experienced by the other wire due to this magnetic field in the nearby wire by making necessary substitution.
Complete step by step answer:
In the given question, we are supplied with the following data:
There are two current carrying wires, which are long and parallel.
We are required to find the force acting between the two parallel current carrying conducting wires.
To begin with, we need to first draw a diagram regarding that. The diagram contains two parallel conducting wires AB and CD, which are separated by some distance
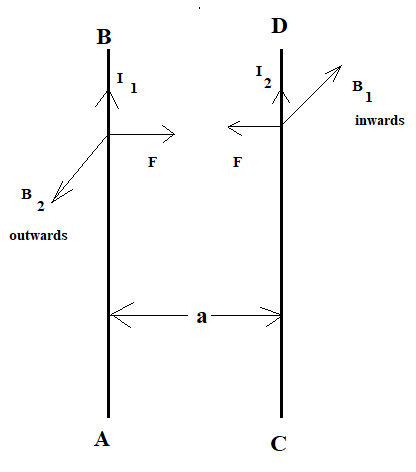
Let us proceed to solve the problem and do the derivation as we are asked.
The two conducting wires are very long.
The magnetic induction which the induced by the current in the first wire i.e. AB, due to the current
Where,
The magnetic field does produce acts perpendicular to the plane of the paper and it is directed inwards. The conducting wire CD is situated in the magnetic field created by the wire AB. So, the force on the part of the wire CD due to the magnetic field present is given by:
Where,
Now, we substitute the equation (1) in equation (2) and we get:
By Fleming’s left-hand rule, this force is directed towards the left.
Similarly, the magnetic induction which the induced by the current in the second wire i.e. CD, due to the current
The magnetic field does produce acts perpendicular to the plane of the paper and it is directed outwards. The conducting wire AB is situated in the magnetic field created by the wire CD. So, the force on the part of the wire AB due to the magnetic field present is given by:
Now, we substitute the equation (3) in equation (4) and we get:
By Fleming’s right-hand rule, this force is directed towards the right.
The two forces defined above, attract one another. Therefore, two parallel wires that carry currents in the same direction attract each other and repel each other if they carry currents in the opposite direction.
For the definition of ampere:
We have,
Where,
Let us take both the current as
Now the equation (5) becomes:
Ampere is defined as the constant current that experiences a force of
Note:
While solving this problem, it is important to remember that the force on one wire is due to the magnetic field of the other wire. Most of the students tend to make mistakes by taking its own magnetic field into account while calculating force on that particular wire. If the currents are moving in the same direction the force attracts and if the currents are moving in the opposite direction then the force repels.
Complete step by step answer:
In the given question, we are supplied with the following data:
There are two current carrying wires, which are long and parallel.
We are required to find the force acting between the two parallel current carrying conducting wires.
To begin with, we need to first draw a diagram regarding that. The diagram contains two parallel conducting wires AB and CD, which are separated by some distance

Let us proceed to solve the problem and do the derivation as we are asked.
The two conducting wires are very long.
The magnetic induction which the induced by the current in the first wire i.e. AB, due to the current
Where,
The magnetic field does produce acts perpendicular to the plane of the paper and it is directed inwards. The conducting wire CD is situated in the magnetic field created by the wire AB. So, the force on the part of the wire CD due to the magnetic field present is given by:
Where,
Now, we substitute the equation (1) in equation (2) and we get:
By Fleming’s left-hand rule, this force is directed towards the left.
Similarly, the magnetic induction which the induced by the current in the second wire i.e. CD, due to the current
The magnetic field does produce acts perpendicular to the plane of the paper and it is directed outwards. The conducting wire AB is situated in the magnetic field created by the wire CD. So, the force on the part of the wire AB due to the magnetic field present is given by:
Now, we substitute the equation (3) in equation (4) and we get:
By Fleming’s right-hand rule, this force is directed towards the right.
The two forces defined above, attract one another. Therefore, two parallel wires that carry currents in the same direction attract each other and repel each other if they carry currents in the opposite direction.
For the definition of ampere:
We have,
Where,
Let us take both the current as
Now the equation (5) becomes:
Ampere is defined as the constant current that experiences a force of
Note:
While solving this problem, it is important to remember that the force on one wire is due to the magnetic field of the other wire. Most of the students tend to make mistakes by taking its own magnetic field into account while calculating force on that particular wire. If the currents are moving in the same direction the force attracts and if the currents are moving in the opposite direction then the force repels.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




