
How do you determine the slope of
Answer
466.2k+ views
Hint:We need to know how to plot a point
Complete step by step solution:
We have to find the slope of
In this question we assume
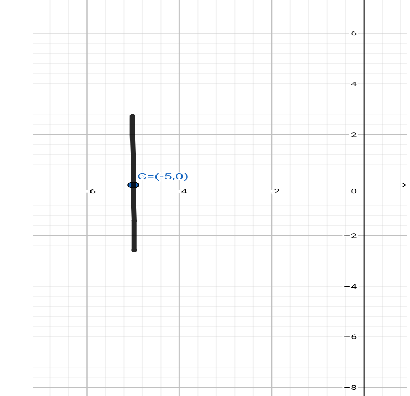
From the graph we can see the given point
So, we get
Here,
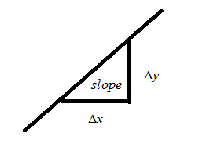
From the graph, we can see the vertical changes but we can’t see the horizontal changes, that is the value of
So, the final answer is,
The slope
Note: We assume
Complete step by step solution:
We have to find the slope of
In this question we assume

From the graph we can see the given point
So, we get
Here,

From the graph, we can see the vertical changes but we can’t see the horizontal changes, that is the value of
So, the final answer is,
The slope
Note: We assume
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

In northern hemisphere 21st March is called as A Vernal class 11 social science CBSE




