Question

Determine what the equation \[\left| z-i \right|=\left| z-1 \right|\] represents given that \[i=\sqrt{-1}\].
(a) The line through the origin with slope \[-1\]
(b) A circle with radius 1
(c) A circle with radius \[\dfrac{1}{2}\]
(d) The line through the origin with slope 1

Answer 1

Hint: In this question, in order to Determine what the equation \[\left| z-i \right|=\left| z-1 \right|\] represents given that \[i=\sqrt{-1}\] we have to first substitute the value of the complex number \[z\] as \[z=x+iy\] in the given equation \[\left| z-i \right|=\left| z-1 \right|\] where \[x\] is the real part of the complex number \[z\] and \[y\] is the it’s imaginary part . Now for any complex number \[z\], modulus of \[z=x+iy\] is defined as \[\left| z \right|=\sqrt{{{x}^{2}}+{{y}^{2}}}\]. Using this in the given equation we will get an equation in variables \[x\] and \[y\]. We have to determine what that equation represents.

Complete step by step answer:
We are given with the equation \[\left| z-i \right|=\left| z-1 \right|\] where \[i=\sqrt{-1}\] and \[z\] is a complex number.
We will now substitute the value of the complex number \[z\] as \[z=x+iy\] in the given equation \[\left| z-i \right|=\left| z-1 \right|\] where \[x\] is the real part of the complex number \[z\] and \[y\] is the imaginary part.
Then we get
\[\left| \left( x+iy \right)-i \right|=\left| \left( x+iy \right)-1 \right|\]
On simplifying the above equation we get
\[\left| x+i\left( y-1 \right) \right|=\left| \left( x-1 \right)+iy \right|\]
On squaring both sides we get,
\[{{\left| x+i\left( y-1 \right) \right|}^{2}}={{\left| \left( x-1 \right)+iy \right|}^{2}}...........(1)\]
We will now find the value of \[{{\left| x+i\left( y-1 \right) \right|}^{2}}\] .
Since we know that for any complex number \[z\], modulus of \[z=a+ib\] is defined as \[\left| z \right|=\sqrt{{{a}^{2}}+{{b}^{2}}}\].
On comparing the values of \[\left| x+i\left( y-1 \right) \right|\] with \[\left| z \right|=\left| a+ib \right|\], we will have
\[a=x\] and \[b=y-1\]
Therefore we have
\[\begin{align}
  & {{\left| x+i\left( y-1 \right) \right|}^{2}}={{\left( \sqrt{{{x}^{2}}+{{\left( y-1 \right)}^{2}}} \right)}^{2}} \\
 & ={{x}^{2}}+{{\left( y-1 \right)}^{2}}.........(2)
\end{align}\]

On comparing the values of \[\left| \left( x-1 \right)+iy \right|\] with \[\left| z \right|=\left| a+ib \right|\], we will have
\[a=x-1\] and \[b=y\]
Therefore we have
\[\begin{align}
  & {{\left| \left( x-1 \right)+iy \right|}^{2}}={{\left( \sqrt{{{\left( x-1 \right)}^{2}}+{{y}^{2}}} \right)}^{2}} \\
 & ={{\left( x-1 \right)}^{2}}+{{y}^{2}}.........(3)
\end{align}\]
Now on substituting the values in equation (2) and equation (3) in equation (1), we will have
\[{{x}^{2}}+{{\left( y-1 \right)}^{2}}={{\left( x-1 \right)}^{2}}+{{y}^{2}}\]
On expanding the above equation we get
\[{{x}^{2}}+{{y}^{2}}-2y+1={{x}^{2}}-2x+1+{{y}^{2}}\]
Now on cancelling out the common factors on both the right hand side and left hand side of the above equation we get
\[-2y=-2x\]
On dividing the above equation by \[-2\], we get
\[y=x\]
Now if we substitute the value \[x=0\] in the equation \[y=x\] we get
\[y=0\]
Also in order to calculate the slope of the line \[y=x\], we have to calculate the derivative \[\dfrac{dy}{dx}\].
Here we have
\[\dfrac{dy}{dx}=1\]
Which is the equation of the line passing the origin \[\left( 0,0 \right)\] with slope 1.

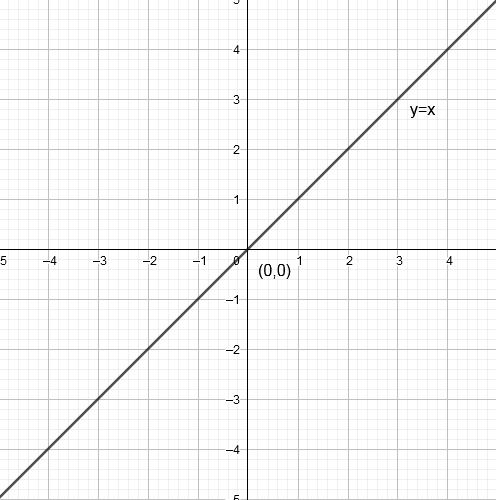
So, the correct answer is “Option D”.

Note: In this problem, when we arrive at an equation in variables \[x\] and \[y\] we have to determine what that equation represents. In our case we have a linear equation in variables \[x\] and \[y\] which is of the form \[y=mx+c\] where \[m\] represents the slope of the line.