
What is the difference between
Answer
443.7k+ views
Hint: In the function
Complete step by step solution:
The trigonometric functions given in the above question are
We can see that both the functions are expressed in terms of the cosine function. The difference between the two is in the first function, the argument is doubled, while in the second function, the cosine of the argument x is doubled.
We know that the cosine is a sinusoidal function whose value varies from
In the function represented in the equation (ii), since the cosine term, whose value varies from
Thus, the range of the function
We can interpret the difference in the two functions by their graphs given below.
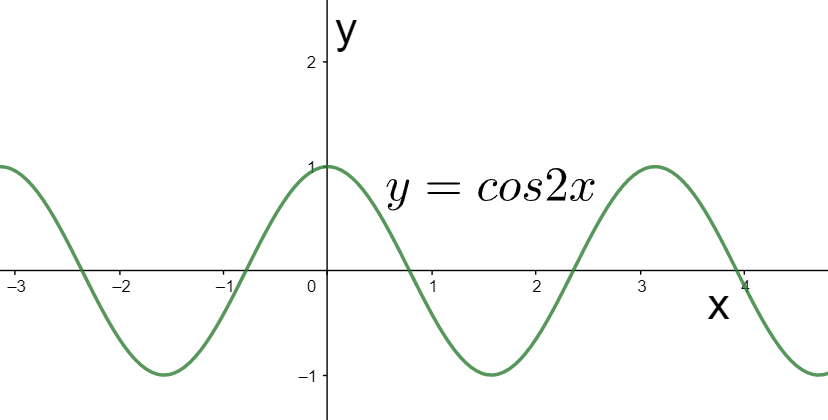
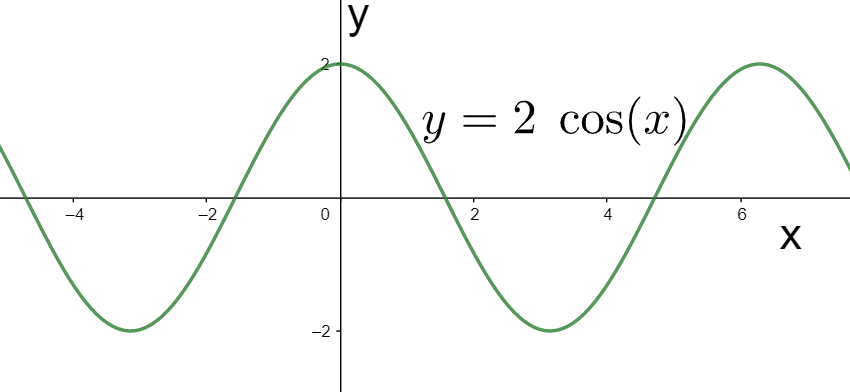
Note: From the graph of the two functions in the above solution we can appreciate that the difference is not only in their ranges, but their periods too. The period of the first function
Complete step by step solution:
The trigonometric functions given in the above question are
We can see that both the functions are expressed in terms of the cosine function. The difference between the two is in the first function, the argument is doubled, while in the second function, the cosine of the argument x is doubled.
We know that the cosine is a sinusoidal function whose value varies from
In the function represented in the equation (ii), since the cosine term, whose value varies from
Thus, the range of the function
We can interpret the difference in the two functions by their graphs given below.


Note: From the graph of the two functions in the above solution we can appreciate that the difference is not only in their ranges, but their periods too. The period of the first function
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Difference between physical and chemical change class 11 chemistry CBSE

Number of oneone functions from A to B where nA 4 and class 11 maths CBSE

What is the z value for a 90 95 and 99 percent confidence class 11 maths CBSE




