
What is the difference between supplementary angles and a linear pair?
Answer
445.2k+ views
Hint: From the given question we are asked to find out the difference between supplementary angles and a linear pair. For solving this question, we will take the help of the definitions of the supplementary angles and a linear pair and explain them briefly. After explaining them briefly, we will find the difference between them and solve the question.
Complete step-by-step solution:
In mathematics, we can use the sum of the measure of angles to categorize angles as different types. That is, if the sum of a pair of angles is equal to certain values, then they fall into the types defined by the sum.
Supplementary angles are defined with respect to the addition of two angles.
If the sum of two angles is said to be
For example,
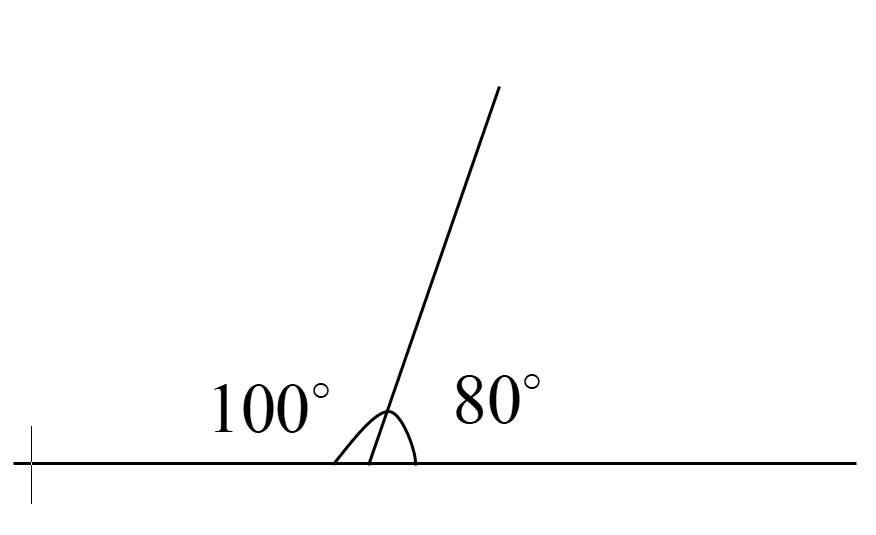
Form the figure, we can say that
Linear pair is a pair of adjacent angles whose noncommon sides form a straight line.
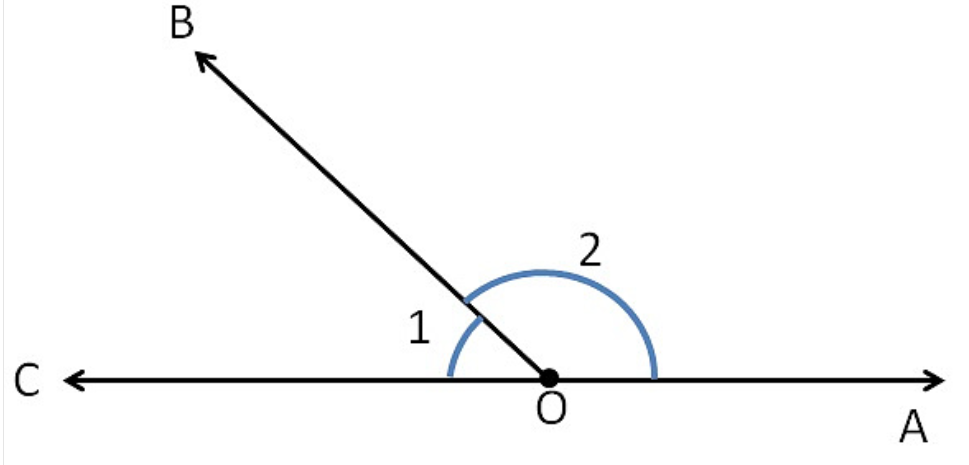
Linear pairs are also said to be pairs of two supplementary angles. But two supplementary angles can or cannot form a linear pair, they have to supplement each other, that is their sum is to be
For example, if there are four linear pairs formed by two intersecting lines. Each pair forms supplementary angles because their sum is
Note: Students should have good knowledge in the concept of angles and it types. We must know the difference between supplementary angles and a linear pair to solve and find the difference between those angles.
Complete step-by-step solution:
In mathematics, we can use the sum of the measure of angles to categorize angles as different types. That is, if the sum of a pair of angles is equal to certain values, then they fall into the types defined by the sum.
Supplementary angles are defined with respect to the addition of two angles.
If the sum of two angles is said to be
For example,

Form the figure, we can say that
Linear pair is a pair of adjacent angles whose noncommon sides form a straight line.

Linear pairs are also said to be pairs of two supplementary angles. But two supplementary angles can or cannot form a linear pair, they have to supplement each other, that is their sum is to be
For example, if there are four linear pairs formed by two intersecting lines. Each pair forms supplementary angles because their sum is
Note: Students should have good knowledge in the concept of angles and it types. We must know the difference between supplementary angles and a linear pair to solve and find the difference between those angles.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





