Question

What is the direction of current density?

Answer 1

Hint: Whenever we hear the word density, the first thing that should strike our mind is the word distribution. For example, words like mass density (just called density in Science), population density, and nuclear density etc. mean that they are talking about the distribution.

Complete step-by-step answer:
The concept of the current density arises from the Ohm’s law expressed in the microscopic form.
Ohm’s law in general, gives us the following expression:
$R = \dfrac{V}{I}$
where R – resistance, V – voltage and I – current.
If we write the microscopic version of the Ohm’s law, we get –
$\rho = \dfrac{E}{J}$
Here, these are corresponding quantities associated with the quantities in the macroscopic or general Ohm’s law:
$\rho $- Resistivity corresponding to Resistance
$E$- electric field corresponding to Voltage
And finally, the quantity J, corresponding to the Current I, is called current density.
Consider the following section of a wire carrying current

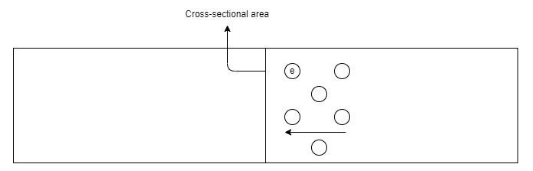
Here, the electrons are travelling through the mentioned cross-sectional area of the wire.
The current density is defined as the amount of charge that passes through the given unit area of cross-section as shown:
If $I$ is the current and A is the cross-sectional area, then:
Current density, $J = \dfrac{I}{A}$
This quantity is a vector whose direction is the area vector perpendicular to the area of cross-section.
The direction of this current density is dependent on the area vector. Since, the area vector points towards the direction of the positive flow or the conventional current direction, the direction of the current density is along that of the conventional current and it is opposite to the direction of the actual current flow.

Note: The current density gives us a feel about the distribution of the current over the unit surface area. The current being a scalar quantity, is therefore, defined as the dot product of the current density and the area vector as shown:
$
  I = \overrightarrow J .\overrightarrow A \\
  I = JA\cos \theta \\
$
Thus, the current will be maximum if the current density vector is along the same direction as that of the area vector.