
Discuss the motion of a charged particle in a uniform magnetic field
Answer
501.6k+ views
1 likes
Hint: The motion of a charged particle in a uniform magnetic field is based on the magnetic Lorentz force. Which states the important features of the magnetic force field and the expression can be written as
Complete step-by-step answer:
Magnetic Lorentz force explains the force F experienced by the particle under the influence of the field. The special features of the force are:
i) The force F on the charge is zero if the charge is at rest. Which means only moving charges are affected by the field.
ii) The force is zero when the direction of motion of the charge is parallel or antiparallel to the field and the force is maximum when the charge is perpendicular to the field.
iii) The force is proportional to the magnitude of charge (q)
iv) It is proportional to the magnetic induction (B)
v) It is also proportional to the speed of charge (v)
vi) The direction of force is opposite for the charges of opposite sign
All these results are summed up as an expression and given as
The magnitude can be written as
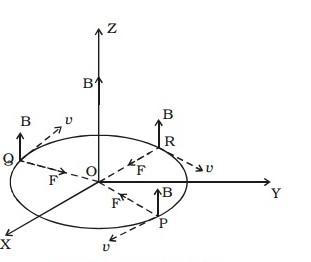
Let us now consider a uniform magnetic field B acting along the Z- axis. If a particle of charge ‘q’ and mass ‘m’ moves in the XY plane. At a point, the velocity of a particle is v.
According to Lorentz force,
As
The Lorentz force provides the centripetal force. Centripetal force is given as
On equating both forces,
From the equation, it is known that radius of the path is proportional to the mass and velocity of the particle.
Note: The equation also provides the angular frequency,
Complete step-by-step answer:
Magnetic Lorentz force explains the force F experienced by the particle under the influence of the field. The special features of the force are:
i) The force F on the charge is zero if the charge is at rest. Which means only moving charges are affected by the field.
ii) The force is zero when the direction of motion of the charge is parallel or antiparallel to the field and the force is maximum when the charge is perpendicular to the field.
iii) The force is proportional to the magnitude of charge (q)
iv) It is proportional to the magnetic induction (B)
v) It is also proportional to the speed of charge (v)
vi) The direction of force is opposite for the charges of opposite sign
All these results are summed up as an expression and given as
The magnitude can be written as

Let us now consider a uniform magnetic field B acting along the Z- axis. If a particle of charge ‘q’ and mass ‘m’ moves in the XY plane. At a point, the velocity of a particle is v.
According to Lorentz force,
As
The Lorentz force provides the centripetal force. Centripetal force is given as
On equating both forces,
From the equation, it is known that radius of the path is proportional to the mass and velocity of the particle.
Note: The equation also provides the angular frequency,
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




