
Do the interior angles of a parallelogram sometimes , always or never sum up to
Answer
386.7k+ views
Hint: In this question, we need to tell whether the interior angles of a parallelogram sometimes , always or never sum up to
Complete step-by-step answer:
Now, let us know the concept and the definition of the parallelogram.
Parallelogram :
A Parallelogram is nothing but a quadrilateral whose two sides are parallel to each other and all the four angles at the vertices are not
Now let us see some of the properties of the parallelogram.
A quadrilateral , which has a pair of parallel opposite sides, then it’s a special polygon, and it is known as the Parallelogram.
1. The opposite sides of the parallelogram are parallel and congruent.
2. The opposite angles of the parallelogram are congruent
3. The consecutive angles of the parallelogram are supplementary in nature.
4. If any one of the angles of the parallelogram is a right angle, then all the other angles of the parallelogram will also be at right angle.
5. The two diagonals of the parallelogram bisect each other.
6. Each diagonal of the parallelogram bisects the parallelogram into two congruent triangles.
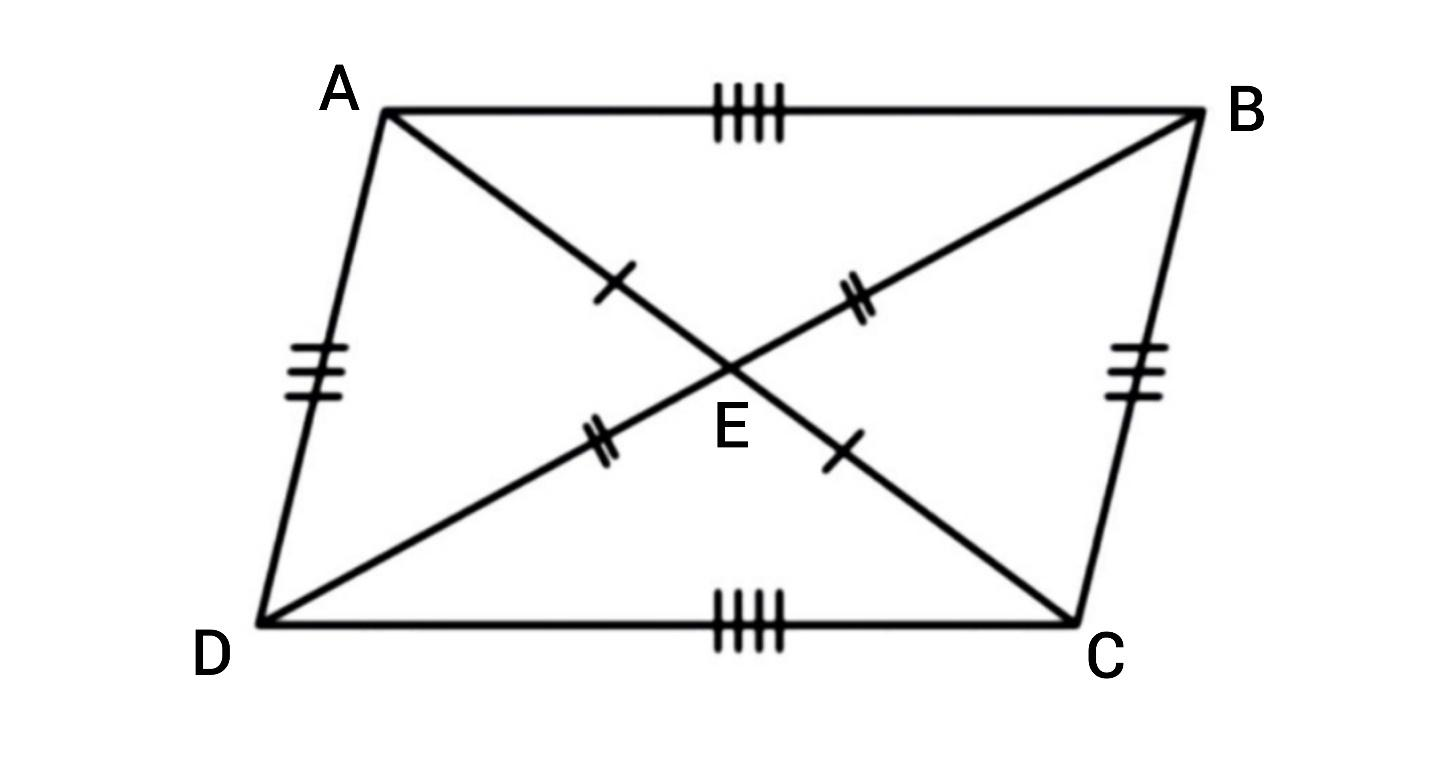
Mathematically, the parallelogram has equivalent opposite interior angles. And also the sum of all four interior angles is always equal to
Thus we can conclude that the sum of the interior angles of a parallelogram is always
Final answer :
The sum of the interior angles of a parallelogram is always
Note: In order to solve these types of questions, we must have a strong grip over the properties of the parallelogram. We also need to know that since the opposite sides of the parallelogram are parallel to each other, and also the diagonals of the parallelogram bisect each other, square is a parallelogram and also the opposite sides of the parallelogram are parallel and diagonals bisect each other, rectangle is also a parallelogram.
Complete step-by-step answer:
Now, let us know the concept and the definition of the parallelogram.
Parallelogram :
A Parallelogram is nothing but a quadrilateral whose two sides are parallel to each other and all the four angles at the vertices are not
Now let us see some of the properties of the parallelogram.
A quadrilateral , which has a pair of parallel opposite sides, then it’s a special polygon, and it is known as the Parallelogram.
1. The opposite sides of the parallelogram are parallel and congruent.
2. The opposite angles of the parallelogram are congruent
3. The consecutive angles of the parallelogram are supplementary in nature.
4. If any one of the angles of the parallelogram is a right angle, then all the other angles of the parallelogram will also be at right angle.
5. The two diagonals of the parallelogram bisect each other.
6. Each diagonal of the parallelogram bisects the parallelogram into two congruent triangles.

Mathematically, the parallelogram has equivalent opposite interior angles. And also the sum of all four interior angles is always equal to
Thus we can conclude that the sum of the interior angles of a parallelogram is always
Final answer :
The sum of the interior angles of a parallelogram is always
Note: In order to solve these types of questions, we must have a strong grip over the properties of the parallelogram. We also need to know that since the opposite sides of the parallelogram are parallel to each other, and also the diagonals of the parallelogram bisect each other, square is a parallelogram and also the opposite sides of the parallelogram are parallel and diagonals bisect each other, rectangle is also a parallelogram.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
The singular of lice is louse A Yes B No class 8 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ounces are in 500 mL class 8 maths CBSE

Advantages and disadvantages of science

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

What led to the incident of Bloody Sunday in Russia class 8 social science CBSE





