
How does Gauss law work inside a conducting sphere?
Answer
468.3k+ views
Hint: An electric conductor has a very large number of charges that moves freely inside the sphere. In the conducting sphere, at all the points inside the sphere, the electric field is zero.
Complete step by step answer:
Let us consider a uniformly charged sphere. The uniformly charged sphere has a radius of
Have the diagram for reference. Consider the point
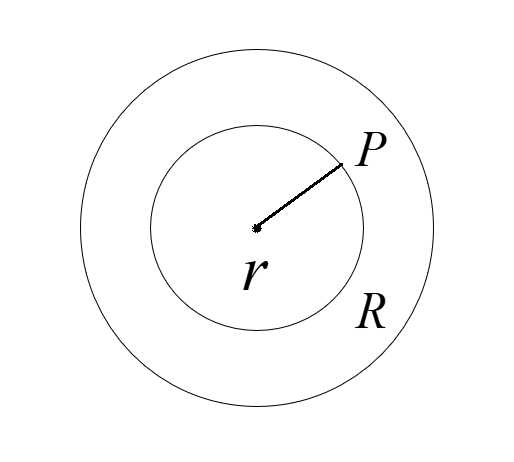
In the diagram, for the points inside the spherical sphere, a spherical gaussian surface is drawn smaller than the spherical sphere
The gaussian surface is drawn around the sphere with the radius. Now let us apply the gauss law. If a charge
Applying the gauss law,
Substitute the value of the area in the formula we get,
Gaussian surface encloses no charge, that is
Zero divided by any number is zero. The value of the electric field will become zero. That is,
From this, we are able to understand that the electric field due to the uniformly charged sphere is zero at all the points inside the shell.
Note:
Suppose if the electric field inside the sphere is not zero then there will be a force acting on the mobile carriers due to the electric field. Due to this, there will be a net motion of the mobile charge carriers that contradicts the conductor being in the electrostatic equilibrium.
Complete step by step answer:
Let us consider a uniformly charged sphere. The uniformly charged sphere has a radius of
Have the diagram for reference. Consider the point

In the diagram, for the points inside the spherical sphere, a spherical gaussian surface is drawn smaller than the spherical sphere
The gaussian surface is drawn around the sphere with the radius. Now let us apply the gauss law. If a charge
Applying the gauss law,
Substitute the value of the area in the formula we get,
Gaussian surface encloses no charge, that is
Zero divided by any number is zero. The value of the electric field will become zero. That is,
From this, we are able to understand that the electric field due to the uniformly charged sphere is zero at all the points inside the shell.
Note:
Suppose if the electric field inside the sphere is not zero then there will be a force acting on the mobile carriers due to the electric field. Due to this, there will be a net motion of the mobile charge carriers that contradicts the conductor being in the electrostatic equilibrium.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




