Question

How does the Fibonacci sequence relate to Pascal's triangle ? \[\]

Answer 1

Hint: We recall the Fibonacci sequence as a sequence of numbers with first two terms 1 and from the third thirds every term will be the sum of previous two terms. We recall Pascal’s triangle as a triangular array containing binomial coefficients ${{\left( x+y \right)}^{n}}$ for $n=0,1,2,3...$. We find the sum entries of diagonals Pascal triangle and relate them with terms of Fibonacci sequence.\[\]

Complete step-by-step solution:
We know that A sequence is defined as the enumerated collection of mathematical objects where repetitions are allowed and order of the numbers matters. The members of the sequence are called terms. Mathematically, a sequence with infinite terms is written as
\[\left( {{x}_{n}} \right)={{x}_{1}},{{x}_{2}},{{x}_{3}},...\]
 We know that a Fibonacci sequence is a sequence of numbers with first two terms 1 and from the third thirds every term will be the sum of previous two terms. We define the terms below:
\[\begin{align}
  & {{x}_{1}}=1 \\
 & {{x}_{2}}=1 \\
 & {{x}_{n}}={{x}_{n-1}}+{{x}_{n-2}}\text{ for all }n\ge 3 \\
\end{align}\]
 It means the third will be ${{x}_{3}}={{x}_{1}}+{{x}_{2}}=1+1=2$, fourth term will be ${{x}_{4}}={{x}_{2}}+{{x}_{3}}=1+2=3$ and so on. So we can write the Fibonacci sequence as
\[1,1,2,3,5,8,13,21...\]
We know that the Pascal triangle is a triangular array containing binomial coefficients. The binomial coefficients are terms appearing in the binomial expansion that ${{\left( x+y \right)}^{n}}$. We put $n=0,1,2,3,...$ to have
\[\begin{align}
  & {{\left( x+y \right)}^{0}}=1 \\
 & {{\left( x+y \right)}^{1}}=1\cdot x+1\cdot y \\
 & {{\left( x+y \right)}^{2}}=1\cdot {{x}^{2}}+2xy+1\cdot y \\
 & {{\left( x+y \right)}^{3}}=1\cdot {{x}^{3}}+3{{x}^{2}}y+3x{{y}^{2}}+1\cdot {{y}^{3}} \\
 & {{\left( x+y \right)}^{4}}=1\cdot {{x}^{4}}+4{{x}^{3}}y+6{{x}^{2}}{{y}^{2}}+4x{{y}^{3}}+1\cdot {{y}^{4}} \\
 & {{\left( x+y \right)}^{5}}=1\cdot {{x}^{5}}+5{{x}^{4}}y+10{{x}^{3}}{{y}^{2}}+10{{x}^{2}}{{y}^{3}}+5x{{y}^{4}}+1\cdot {{y}^{5}} \\
\end{align}\]
So we can write the Pascal triangle up to 6 rows \[\]

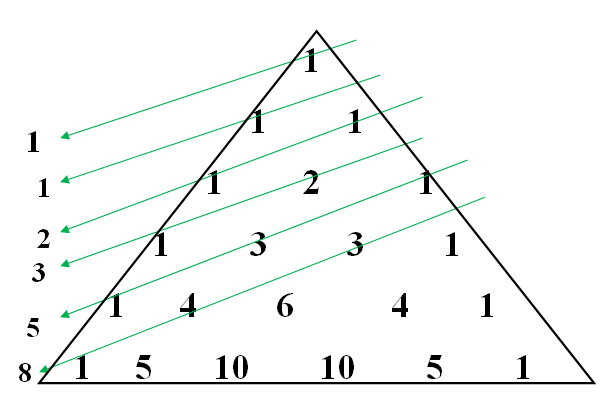
Let us observe the diagonal of the Pascal triangle. We see that in the first diagonal we have 1 and in the second diagonal 1. We see in the third diagonal we have 1 and 1 whose sum is $1+1=2$. We have in the fourth diagonal $1,2$ whose sum is $1+2=3$ and in the fourth diagonal we have $1+3+1=5$. We have in fifth diagonal we have $1+3+4=8$. So we have the sums in diagonals as
\[1,1,2,3,5,8,...\]
So we see that the sum of entries in ${{n}^{\text{th}}}$ diagonal is equal to ${{n}^{\text{th}}}$ terms of the Fibonacci sequence.

Note: We note that the coefficients in the binomial expansion of two terms is given by ${{\left( x+y \right)}^{n}}={}^{n}{{C}_{0}}{{x}^{n}}y+{}^{n}{{C}_{1}}{{x}^{n-1}}y+...+{}^{n}{{C}_{n}}{{x}^{0}}{{y}^{n}}$ and the binomial coefficients are given by ${}^{n}{{C}_{0}},{}^{n}{{C}_{1}},...,{}^{n}{{C}_{n}}$. We can evaluate a binomial coefficient using the combinatorial formula${}^{n}{{C}_{r}}=\dfrac{n\left( n-1 \right)...\left( n-r+1 \right)}{r\left( r-1 \right)...1}$. We also see that Pascal’s triangle sum of two terms is written just in the middle of below them.