
Draw a circle of diameter 7 cm and construct tangents at the ends of the diameter.
Answer
483k+ views
1 likes
Hint: We will draw the circle using a compass. First, we will draw its diameter using a scale. Then, we will construct a perpendicular bisector at both ends of its diameter. These bisectors will be the tangents to the circle.
Formulas used:
We will use the formula
Complete step-by-step answer:
We know that the diameter of a circle is 2 times its radius.
Now, we will calculate the radius of the circle.
Dividing both side by 2, we get
We have calculated that the radius of the circle is 3.5 cm
First, we will draw a circle of radius 3.5 cm. We will take a scale and open the compass 3.5 cm. We will choose a centre for the circle and construct it. We will also draw the diameter of the circle by making a line passing through its centre:
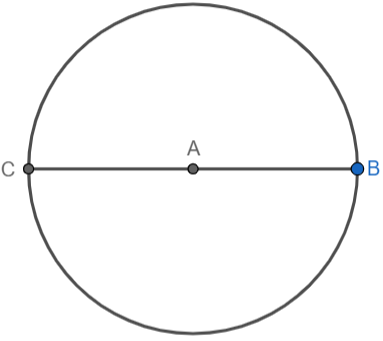
We know that tangents are perpendicular to the radius at the point of contact. We will construct right angles at points C and B. We will take C as the centre and construct a small semi-circle using a compass:
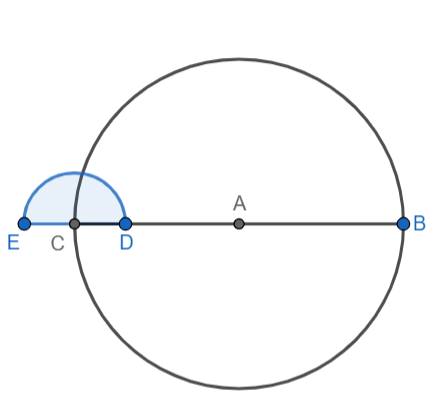
We will take point D as centre and keep the same radius used for drawing the semi-circle. We will draw an arc, intersecting the semicircle at point H. This arc will represent an angle of
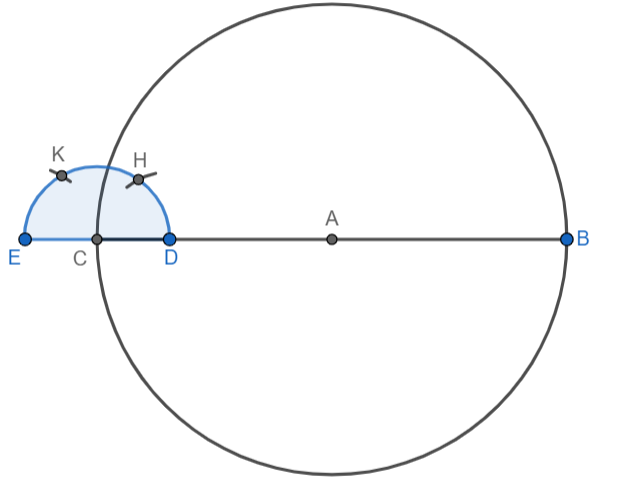
Taking the same radius, we will draw an arc with H as centre and an arc with K as centre. We will label the point of intersection of these arcs as P:

We will join points P and C and extend the line segment. This line will be the tangent to the circle at point C:
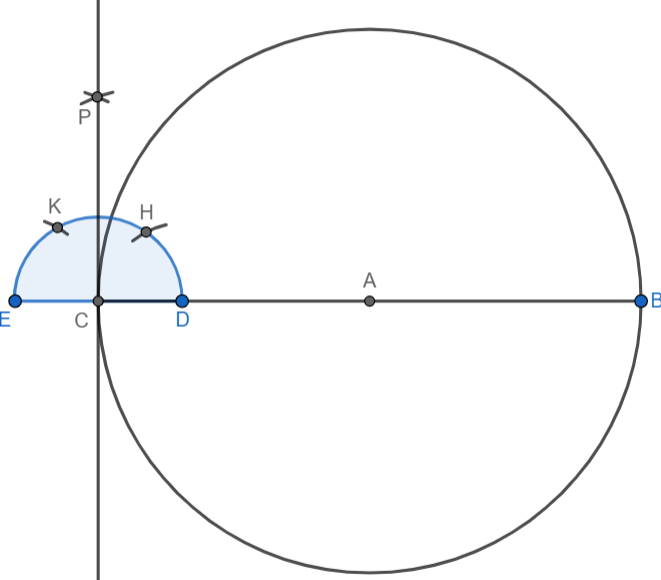
We will draw a tangent at point B using the same procedure as mentioned above:
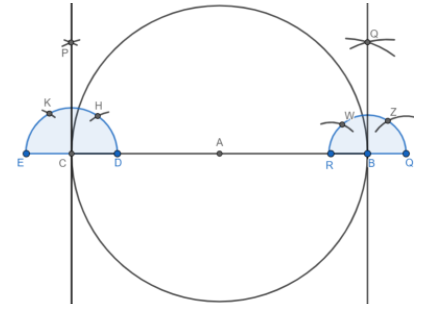
QB is the tangent to the circle at point B.
Note: We must be careful that the radius taken to construct angles of
Formulas used:
We will use the formula
Complete step-by-step answer:
We know that the diameter of a circle is 2 times its radius.
Now, we will calculate the radius of the circle.
Dividing both side by 2, we get
We have calculated that the radius of the circle is 3.5 cm
First, we will draw a circle of radius 3.5 cm. We will take a scale and open the compass 3.5 cm. We will choose a centre for the circle and construct it. We will also draw the diameter of the circle by making a line passing through its centre:

We know that tangents are perpendicular to the radius at the point of contact. We will construct right angles at points C and B. We will take C as the centre and construct a small semi-circle using a compass:

We will take point D as centre and keep the same radius used for drawing the semi-circle. We will draw an arc, intersecting the semicircle at point H. This arc will represent an angle of

Taking the same radius, we will draw an arc with H as centre and an arc with K as centre. We will label the point of intersection of these arcs as P:

We will join points P and C and extend the line segment. This line will be the tangent to the circle at point C:

We will draw a tangent at point B using the same procedure as mentioned above:

QB is the tangent to the circle at point B.
Note: We must be careful that the radius taken to construct angles of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





