Question

Draw a line segment of length 10 cm and bisect it. Further bisect one of the equal parts and measure its length.

Answer 1

Hint: A line segment is a part of a line that connects two pints. It has a length. We also know that a perpendicular bisector is a line segment which intersects another line perpendicularly and divides it into two equal parts. Using these definitions we can solve this problem. To get a perpendicular bisector we need a line segment.

Complete step-by-step answer:
Let’s first draw a line segment of length 10 cm.
We follow these steps.
 \[ \bullet \] Draw a line ‘L’
Mark points ‘S’ on it.

 \[ \bullet \] Since length is 10cm, we measure 10 cm using ruler and we mark ‘T’ at exactly 10 cm from point ‘S’.

Thus we have a line segment ‘ST’ of length 10cm.
Now to construct the perpendicular bisector, we follow these steps.
 \[ \bullet \] Since we have a line segment ‘ST’. Take ‘A’ as a centre and radius more than half of the length of AB, draw two arcs, one on either side of ‘ST’.

 \[ \bullet \] Similarly take ‘T’ as centre and the same length taken in the previous step, draw two arcs, cutting the previously drawn arcs. We get points ‘A’ and ‘C’.
 

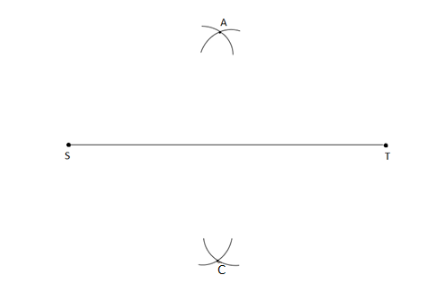
 \[ \bullet \] In the final steps we join the points ‘A’ and ‘C’ by a straight line. This straight line intersects the line ‘ST’ at ‘P’.

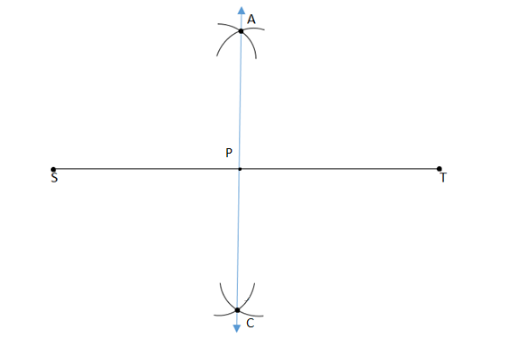
 \[ \bullet \] With ‘P’ as a centre and ‘T’ as another centre with the same radius as in the above step draw arcs intersecting the arcs at ‘D’ and ‘E’. (Follow the same steps as above). And draw the line segment joining ‘D’ and ‘E’. which intersect the line ‘PT’ at ‘B’.

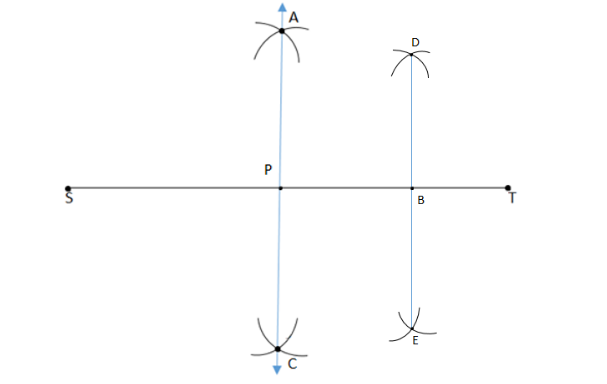
Since we can see that the length of ‘ST’ is 10 cm and point ‘P’ bisects the line so we have length of PT=SP=5 cm. again the point B bisect P we have length PB=BT=2.5 cm. (We measure this using a ruler.)
So, the correct answer is “2.5 cm”.

Note: In drawing the bisector line, we need to construct arcs and we need to take more than half of the length of line ‘ST’ while drawing an arc. In this problem we have a length of ST that is 10 cm and half of it is 5 cm. In both cases while taking ‘S’ and ‘T’ as a centre for drawing an arc we take the radius length more than 5 cm.