Question

Draw a pie chart for the following data:

Item of expenditure	Food	Health	Clothing	Education	Savings
Amount spent (in rupees)	3750	1875	1875	1200	7500

Answer 1

Hint: Pie chart is drawn in a circle. So, first, we convert each item of expenditure into angle assuming the total angle in a circle \[{{360}^{0}}\] corresponds to income. Conversion of angle corresponding to the item is calculated by using the formula
\[\text{angle}=\dfrac{\text{amount spent on that item}}{\text{total income}}\times {{360}^{0}}\]
After finding the angle for each item of expenditure we divide the circle corresponds to that angle and gives a name to each part.

Complete step-by-step solution:
Let us assume\[I\] be the income.
Let us find the total income by adding the amount spent on each item as
\[\begin{align}
  & \Rightarrow I=3,750+1,875+1,875+1,200+7,500 \\
 & \Rightarrow I=16,200 \\
\end{align}\]
So, the total income is 16,200 rupees.
Now, let us calculate the angle for each item of expenditure by using the formula
\[\text{angle}=\dfrac{\text{amount spent on that item}}{\text{total income}}\times {{360}^{0}}\]
(i) Let us calculate angle for food
By using the above formula we get
\[\begin{align}
  & \Rightarrow \text{angle of food}=\dfrac{3,750}{16,200}\times {{360}^{0}} \\
 & \Rightarrow \text{angle of food}=83.34\simeq {{83}^{0}} \\
\end{align}\]
(ii) Let us calculate angle for health
By using the above formula we get
\[\begin{align}
  & \Rightarrow \text{angle of health}=\dfrac{1,875}{16,200}\times {{360}^{0}} \\
 & \Rightarrow \text{angle of clothing}=41.67\simeq {{42}^{0}} \\
\end{align}\]
(iii) Let us calculate angle for clothing
By using the above formula we get
\[\begin{align}
  & \Rightarrow \text{angle of clothing}=\dfrac{1,875}{16,200}\times {{360}^{0}} \\
 & \Rightarrow \text{angle of clothing}=41.67\simeq {{42}^{0}} \\
\end{align}\]
(iv) Let us calculate angle for education
By using the above formula we get
\[\begin{align}
  & \Rightarrow \text{angle of education}=\dfrac{1,200}{16,200}\times {{360}^{0}} \\
 & \Rightarrow \text{angle of education}=26.67\simeq {{27}^{0}} \\
\end{align}\]
(v) Let us calculate angle for savings
By using the above formula we get
\[\begin{align}
  & \Rightarrow \text{angle of savings}=\dfrac{7,500}{16,200}\times {{360}^{0}} \\
 & \Rightarrow \text{angle of savings}=166.34\simeq {{166}^{0}} \\
\end{align}\]
Now, by making these angles as parts in a circle we get a pie chart as

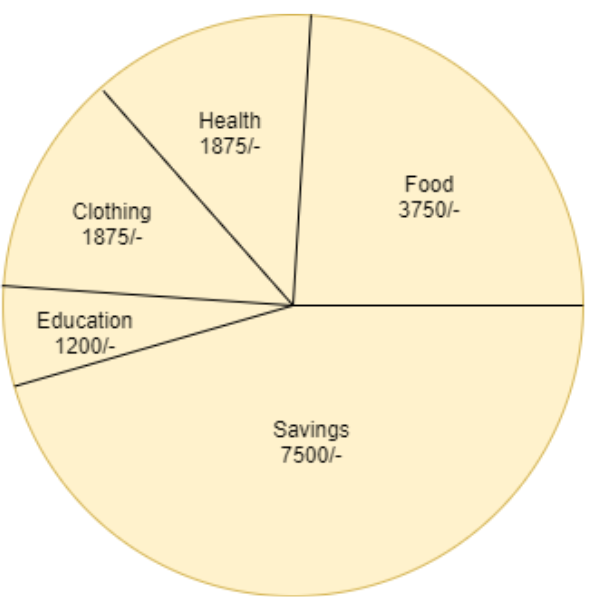
Note: Students may do mistake in following points
(i) We need to mention each part with its name.
(ii) Give each part to a certain design to avoid confusion
(iii) Angle should not be mentioned in the pie diagram but you can mention the amount spent.
Above mentioned points need to be taken care of while drawing the pie chart.