
Draw a plot showing the variation of (i) electric field (E) and (ii) electric potential (V) with the distance r for a point charge Q.
Answer
500.4k+ views
Hint: When a point charge particle is placed at a point, it creates a force field around it. This is called the electric field (E). Now, when you move a point unit charge under this field from infinity to a particular point, the work done by the particle is called electric potential. Electric potential difference between two points is equal to work done by the unit charge point particle moving one point to another.
Complete step by step answer:
A point particle with charge Q placed at origin. From Coulomb’s law, the electric field of the charge will be,
Now, according to definition above, the electric potential will be,
Now, plotting these two functions with respect to r, we get
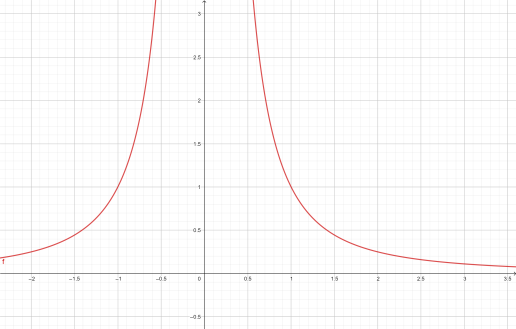
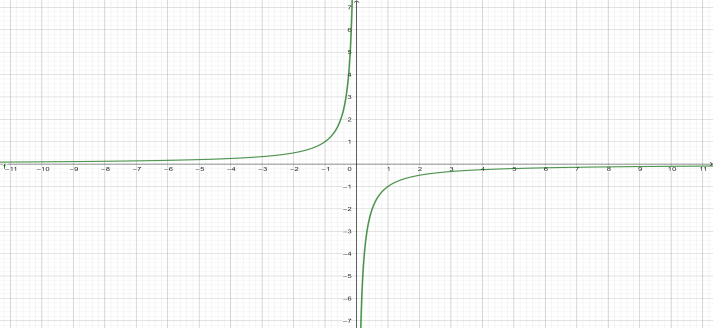
Fig-1: Electric field Fig-2: Electric potential
If we closely look at it we will see, in both cases if we take r close to origin value of potential and field goes very high. And we are ignoring the negative section of the plot, since in polar coordinates only positive values of r exist.
Note:
The S.I unit of the electric field is Newton/coulomb (N/C) which is the same as volt/meter (V/m). The S.I unit of electric potential is volt (V). In a cgs unit or Gaussian unit, the unit of electric field and electric potential is stat volt/stat coulomb and stat volt.
Complete step by step answer:
A point particle with charge Q placed at origin. From Coulomb’s law, the electric field of the charge will be,
Now, according to definition above, the electric potential will be,
Now, plotting these two functions with respect to r, we get


Fig-1: Electric field Fig-2: Electric potential
If we closely look at it we will see, in both cases if we take r close to origin value of potential and field goes very high. And we are ignoring the negative section of the plot, since in polar coordinates only positive values of r exist.
Note:
The S.I unit of the electric field is Newton/coulomb (N/C) which is the same as volt/meter (V/m). The S.I unit of electric potential is volt (V). In a cgs unit or Gaussian unit, the unit of electric field and electric potential is stat volt/stat coulomb and stat volt.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




