
Draw phasor diagram for a series LCR circuit with alternating voltage source, determine the expression for the impedance of the circuit.
Answer
506.4k+ views
1 likes
Hint: For getting the phasor diagram for the given conditions we will first apply the kvl across the circuit and then from the equations obtained from the kvl we will also get the expressions for the series impedance.
Complete step-by-step answer:
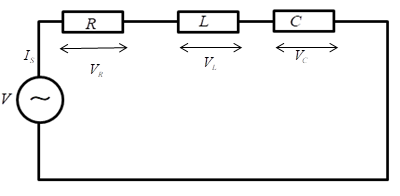
The voltage across each element in the circuit is given by
Let
Applying KVL in the above circuit
Where
Now according to Ohm’s law
V = IR
Now
The voltage across resistor
The voltage across inductor
The voltage across capacitor
Substituting these values in the equation (1)
As we know the current leads the voltage by 90 degree in capacitor and lags by 90 degree in case of inductor.
So, taking the magnitude of above equation and assume
Let
Z is known as impedance.
The phasor diagram of the given circuit is shown below.
The resultant vector will be obtained by adding the two vectors
Because the two vectors are 180 degree apart as shown in the below figure
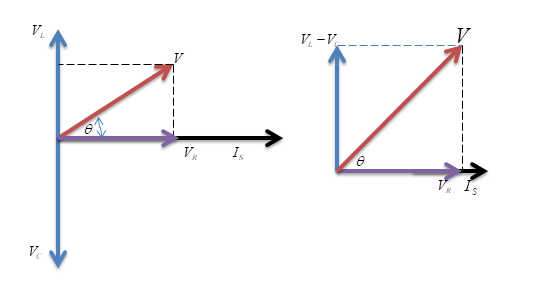
Note – While drawing the phasor diagram, the final reactive voltage must be positive in value therefore take the smallest voltage of the reactive element and subtract it from the largest voltage of the reactive element in the phasor diagram and then take the resultant using the Pythagoras theorem. The current vector is always in phase with the resistance voltage.
Complete step-by-step answer:

The voltage across each element in the circuit is given by
Let
Applying KVL in the above circuit
Where
Now according to Ohm’s law
V = IR
Now
The voltage across resistor
The voltage across inductor
The voltage across capacitor
Substituting these values in the equation (1)
As we know the current leads the voltage by 90 degree in capacitor and lags by 90 degree in case of inductor.
So, taking the magnitude of above equation and assume
Let
Z is known as impedance.
The phasor diagram of the given circuit is shown below.
The resultant vector will be obtained by adding the two vectors
Because the two vectors are 180 degree apart as shown in the below figure

Note – While drawing the phasor diagram, the final reactive voltage must be positive in value therefore take the smallest voltage of the reactive element and subtract it from the largest voltage of the reactive element in the phasor diagram and then take the resultant using the Pythagoras theorem. The current vector is always in phase with the resistance voltage.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




