
Draw the graph of
Answer
504k+ views
1 likes
Hint: For drawing the graph of
Complete step-by-step answer:
We have to draw the plot for the function
Now, before we proceed to plot the curve we should know some fundamental concepts of functions and necessary conditions for a function, so that its inverse exists.
One-one Functions:
A function
Onto Functions:
A function
Now, functions which are both on-one and onto then, such functions are called as bijective functions. And in bijective functions, the number of elements in the domain and co-domain should be equal.
Now, as we know that corresponding to every bijection (one-one-onto function)
Thus, we have
Now, as we know that trigonometric functions are periodic functions and hence, in general, all trigonometric functions are not bijections. Consequently, their inverse does not exist. However, if we restrict the domains and co-domains, they can be bijective functions and we can obtain their inverses.
Now, before we draw a plot for the function
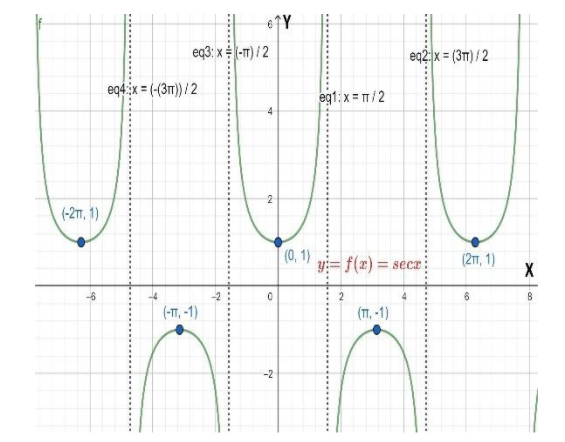
In the above figure, the general plot of the function
Now, consider the function
Now, it is evident from the graph of
Now, the inverse of
Now, in order to plot,
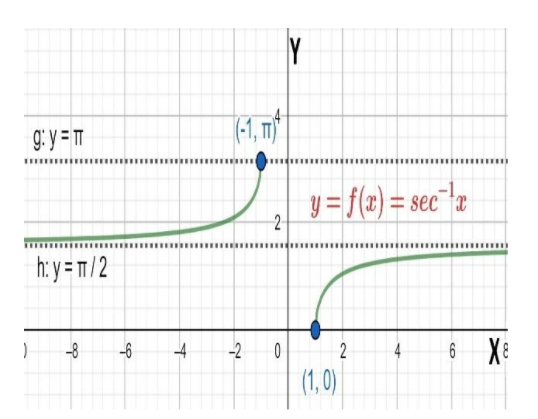
Now, the above plot is the required plot of the function
Note: Here, the student should first understand how we are making a trigonometric function bijective. Then, we should remember the standard conventions that are considered for the function
Complete step-by-step answer:
We have to draw the plot for the function
Now, before we proceed to plot the curve we should know some fundamental concepts of functions and necessary conditions for a function, so that its inverse exists.
One-one Functions:
A function
Onto Functions:
A function
Now, functions which are both on-one and onto then, such functions are called as bijective functions. And in bijective functions, the number of elements in the domain and co-domain should be equal.
Now, as we know that corresponding to every bijection (one-one-onto function)
Thus, we have
Now, as we know that trigonometric functions are periodic functions and hence, in general, all trigonometric functions are not bijections. Consequently, their inverse does not exist. However, if we restrict the domains and co-domains, they can be bijective functions and we can obtain their inverses.
Now, before we draw a plot for the function

In the above figure, the general plot of the function
Now, consider the function
Now, it is evident from the graph of
Now, the inverse of
Now, in order to plot,

Now, the above plot is the required plot of the function
Note: Here, the student should first understand how we are making a trigonometric function bijective. Then, we should remember the standard conventions that are considered for the function
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




