
Draw the graph of
Answer
516k+ views
Hint: To show the continuity of a function, we should ensure that it exists at all points and there are no breaks or sharp edges on the graph of that function. To check the differentiability of a function
Complete step by step answer:
The graphs of the two functions are-
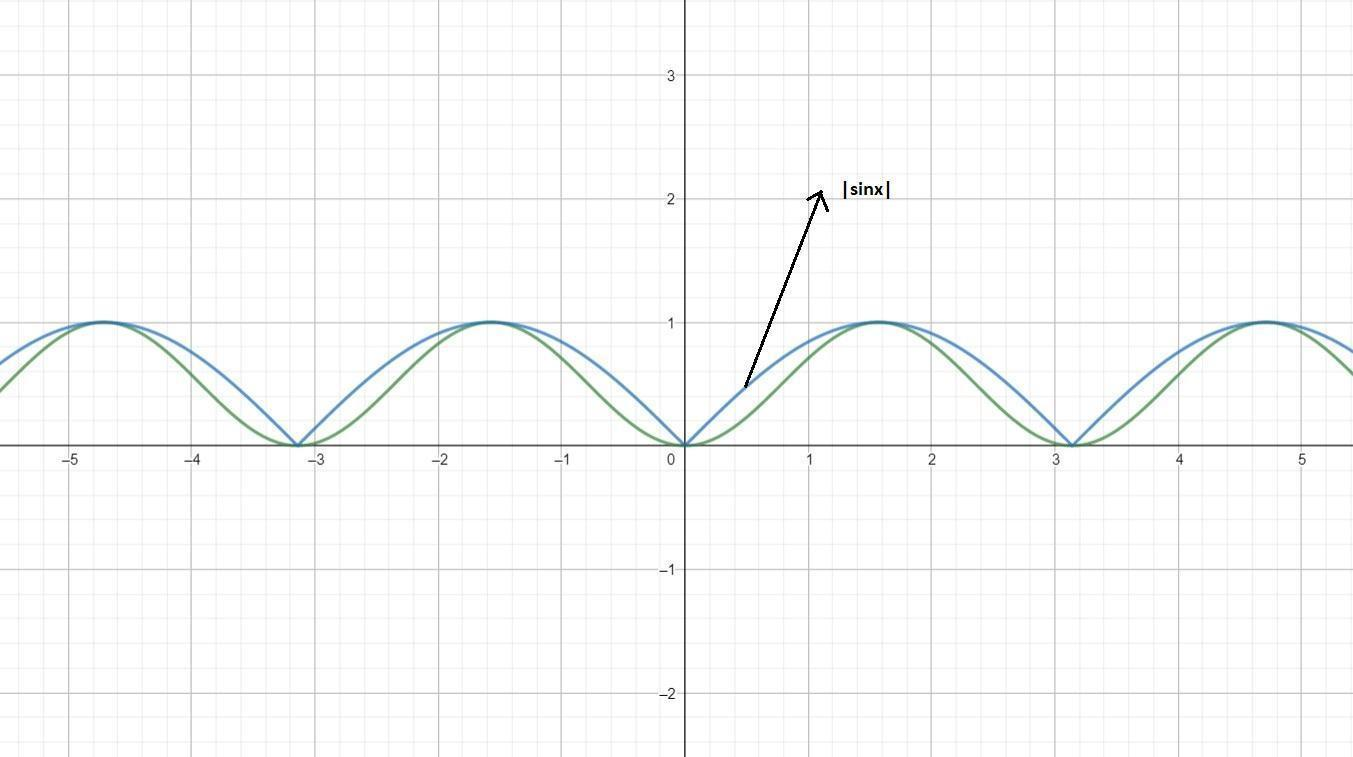
Here the the graph which is inside is
Since
Since
This is the required answer.
Note: Initially when looking at the graph, it seems that both the functions are perfectly smooth, but it is not right. Due to the presence of modulus function,
Complete step by step answer:
The graphs of the two functions are-

Here the the graph which is inside is
Since
Since
This is the required answer.
Note: Initially when looking at the graph, it seems that both the functions are perfectly smooth, but it is not right. Due to the presence of modulus function,
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




