
Draw the graph of the smallest integer function f(x) = [x]
Answer
529.2k+ views
1 likes
Hint: Smallest integer function will give the same number if the number is an integer but it will give integer to the right of the number if the given number is in decimal form (no integer). Ex: [5.3] = 6, [6] = 6. Use this definition and define the smallest integer function mathematically from the domain of
Complete step-by-step answer:
Smallest integer function is a function which takes all the values
So, let us find the smallest integer value of 5.3 i.e. we have to find the value of [5.3].
So, represent it on number line as

Now, we can observe that the integer just right to 5.3 is 6, it means smallest integer value of 5.3 is 6 i.e. [5.3] = 6
Example:
i) [0.5] = 1
ii) [2.3] = 3
iii) [-3.2] = -3
iv) [4] = 4
v) [-5] = -5
Smallest integer function will give the same number if the number is an integer but it will give integer to the right of the number if the number is of decimal form (not integer). Let us understand this definition with examples: Now, we can proceed, for drawing the graph of y = [x].
Let us define the smallest integer function for smaller ranges of domains.
So, we know, If
Above results are given based on the definition of smallest integer function as it gives the right integer by the given number in domain on the number line (in case when number is not an integer) and if domain is an integer then we get the same integer in range as well. So, let us draw the graph with the help of the above results. So, we need to draw line y = 1 in domain of
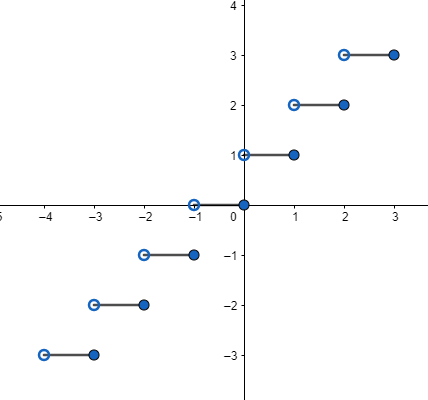
Where ‘0’ (white circle) means x = 0, x = 1, x = 2 and others will not lie on lines y = 1, y = 2, y = 3………. Respectively, ‘0’ (dark circle) means x = 0, x = 1, x = 2………… will lie on lines y = 0, y = 1, y = 2…….. respectively. It’s just a representation for excluding or including any value.
Note: One may take left side integers on the number line by putting the decimal numbers to the given smallest integer function, which is wrong. We take left side integers in case of greatest integer function.
Complete step-by-step answer:
Smallest integer function is a function which takes all the values
So, let us find the smallest integer value of 5.3 i.e. we have to find the value of [5.3].
So, represent it on number line as

Now, we can observe that the integer just right to 5.3 is 6, it means smallest integer value of 5.3 is 6 i.e. [5.3] = 6
Example:
i) [0.5] = 1
ii) [2.3] = 3
iii) [-3.2] = -3
iv) [4] = 4
v) [-5] = -5
Smallest integer function will give the same number if the number is an integer but it will give integer to the right of the number if the number is of decimal form (not integer). Let us understand this definition with examples: Now, we can proceed, for drawing the graph of y = [x].
Let us define the smallest integer function for smaller ranges of domains.
So, we know, If
Above results are given based on the definition of smallest integer function as it gives the right integer by the given number in domain on the number line (in case when number is not an integer) and if domain is an integer then we get the same integer in range as well. So, let us draw the graph with the help of the above results. So, we need to draw line y = 1 in domain of

Where ‘0’ (white circle) means x = 0, x = 1, x = 2 and others will not lie on lines y = 1, y = 2, y = 3………. Respectively, ‘0’ (dark circle) means x = 0, x = 1, x = 2………… will lie on lines y = 0, y = 1, y = 2…….. respectively. It’s just a representation for excluding or including any value.
Note: One may take left side integers on the number line by putting the decimal numbers to the given smallest integer function, which is wrong. We take left side integers in case of greatest integer function.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




