
How do you draw the slope field of the differential equation
Answer
468.3k+ views
Hint: A slope field is a visual representation of a differential equation of the form
Complete step by step answer:
If we have given a differential equation in x and y, we can draw a segment with
we have to substitute values of x and y into our differential equation. The result will be the value of
Consider a case
Then the differential equation will be
This means
It is shown in the below figure.
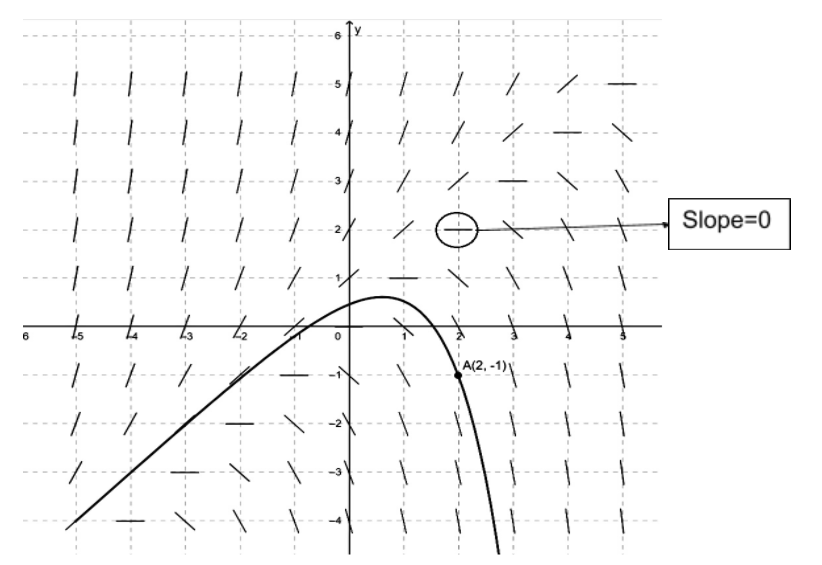
We can say that at every point where
Similarly, by taking other points and plotting their slopes at their respective points gives us the slope field graph.
In this way we can plot the slope field of any differential equation.
Note: In order to solve these types of problems, we must have enough knowledge over slope field equations, differential equations. We may use pencil and paper and draw at each point a little line with inclination representing the value calculated at that point.
Complete step by step answer:
If we have given a differential equation in x and y, we can draw a segment with
we have to substitute values of x and y into our differential equation. The result will be the value of
Consider a case
Then the differential equation will be
This means
It is shown in the below figure.

We can say that at every point where
Similarly, by taking other points and plotting their slopes at their respective points gives us the slope field graph.
In this way we can plot the slope field of any differential equation.
Note: In order to solve these types of problems, we must have enough knowledge over slope field equations, differential equations. We may use pencil and paper and draw at each point a little line with inclination representing the value calculated at that point.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




