Question

Draw two squares of different sides. Can you say they are similar? Explain. Find the ratio of their perimeters and areas. What do you observe?

Answer 1

Hint: Two squares with different side lengths.
We will make any two squares with two different side lengths. After that, we will take the ratio of the corresponding sides of the two different squares to check the similarity of these two squares.
We will use the formulas of the perimeter and area of the triangle to find the area and perimeter.


Complete step by step solution:
Let us draw two squares ABCD and EFGH of sides 2 cm and 4 cm.
Steps to draw a square:
1.Draw a side of the square using a ruler. Keep track of the length of this side so you can make all four sides the same length.
2.Considering the side drawn in the previous step as one line segment with two ends, construct a right angle on each end of it. Thus, the endpoint of the side drawn in the previous step will also be the two vertices of these right angles.
3.Mark a point on each of the new drawing arms (of the two right angles), at a distance (measured from each vertex of the right angle) which is the same as the length of the sides drawn initially. Join these two points.

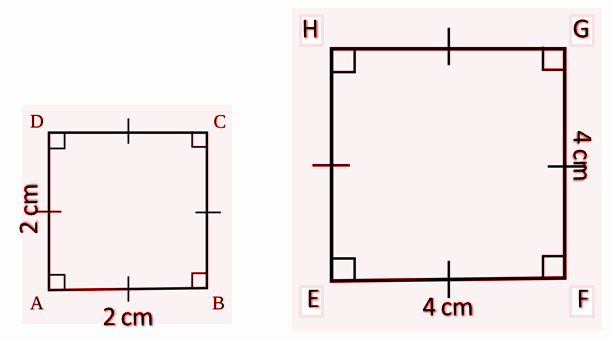
As all the sides are in proportion
\[\dfrac{EF}{AB}=\dfrac{FG}{BC}=\dfrac{GH}{CD}=\dfrac{HE}{DA}=\dfrac{4}{2}=\dfrac{2}{1}\]
And all the pairs of corresponding angles are \[{{90}^{\circ }}\] .thus they are similar. Having corresponding angles at \[{{90}^{\circ }}\] means that all the corner points are perpendicular to each other.
So square \[ABCD\sim \text{square}\,\,EFGH\]
The perimeter of Square \[ABCD=4\times \text{length of sides=}4\times 2=8\,\text{centimeter}\text{.}\]
The perimeter of Square \[EFGH=4\times \text{length of sides=}4\times 4=16\,\text{centimeter}\]
The ratio of their perimeters \[\text{=}\dfrac{8}{16}=\dfrac{1}{2}\]
The ratio of their perimeters is the same as the ratio of their corresponding sides.

Area of \[ABCD=\text{length}\times \text{breadth=2}\times 2=4\,\text{centimeter square}\text{.}\]
Area of\[EFGH=\text{length}\times \text{breadth=4}\times 4=16\,\text{centimeter square}\]
The ratio of their area \[\text{=}\dfrac{4}{16}=\dfrac{1}{4}=\dfrac{{{1}^{2}}}{{{2}^{2}}}\]
The ratio of their areas is the same as the ratio of squares of the corresponding sides.



Note: First draw two squares and make sure their lengths are different. The drawing of the square should be done carefully.
we can also take different values for the length of each side since the ratio of the perimeter will always be the same as the ratio of the corresponding sides. And the Ratio of their areas will always be the same as the ratio of squares of the corresponding sides.
Construction of \[{{90}^{\circ }}\]should be such that they are accurate. We can use a compass or protractor.