Question

Draw, wherever possible, a rough sketch of:
A quadrilateral with line symmetry but not a rotational symmetry of order more than 1.

Answer 1

Hint: The above question demands that there should be no rotational symmetry. It means that when a quadrilateral is rotated till the angle of \[{{360}^{\circ }}\], there should not be any angle of rotation at which the quadrilateral resembles the quadrilateral in an unrotated position. Also the question demands that there should be at least one line in the quadrilateral with respect to which it is symmetric. So, now we are going to see what type of quadrilateral can be drawn.

Complete step-by-step answer:
Thus, we have to draw a quadrilateral which satisfies both the condition given in question. Thus, the quadrilateral which satisfies these conditions is a ‘kite’. Following is a figure of the kite.

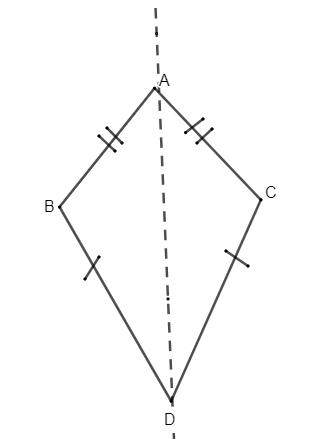
Thus, we can see that a kite is a type of quadrilateral which has no rotational symmetry; that is when it is rotated in a full circle, it never comes in a position which is the same as the position of an unrotated kite. Hence, we can say that a kite is a quadrilateral which has rotational symmetry order equal to 1. Thus, we have to check for the first condition. We can clearly see that the axis \[x-y\] is acting as a mirror for one portion of the kite. So, \[x-y\] line is the line of symmetry and hence we can say that a kite has a line of symmetry.
Thus a kite is satisfying both the conditions given in question.

Note: There is an exception to the kites. When all the sides of the kite become equal, that is when \[AB=BD=DC=CA\], then it becomes rhombus and as we know that a rhombus has both line symmetry and rotational symmetry, we cannot draw rhombus in this case.