Question

Edge length of a cube is 400pm. Its body diagonal (in pm) would be:
[A] 566
[B] 600
[C] 500
[D] 693

Answer 1

HINT: Body diagonal of a cube uses the diagonal drawn between two vertices which are not on the same face. To solve this use the formula of the diagonal of a cube that is the square root of the sum of squares of length, breadth and height of a cube. For a cube all these will be equal so use the edge length to find the required diagonal.

COMPLETE STEP BY STEP SOLUTION: We know that body diagonal of any polyhedron is a line connecting two vertices that do not lie on the same face.
Pictorially we can represent it as -

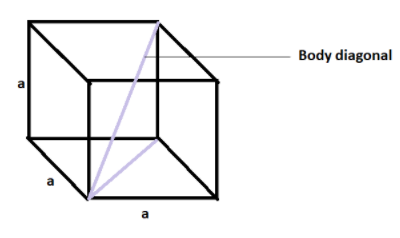
Where ‘a’ is the edge length which is equal for all the edges as it is a cube.
Now, we know that we can write the diagonal of a cuboid as $\sqrt{l^2+b^2+h^2}$ , where l is the length of the cuboid, b is the breadth of the cuboid and h is the height of the cuboid.
In the given question we have a cube. Therefore, length, breadth and height all are equal to each other.
Therefore, we can write that the diagonal of the cube will be $\sqrt{3a^2}$ that is $\sqrt{3}a$ where ‘a’ is the edge length.
Here, the edge length is given to us as 400pm.
Therefore, we can write that the body diagonal of the cube will be $\sqrt{3}\times 400$ pm = 692.82 pm.
We can understand from the above calculation that the body diagonal of the cube is 692.82 pm which is almost equal to 693 pm.

Therefore, the correct answer is option [D] 693.

NOTE: Using the body diagonal of a cubic unit cell and the radius of the cations and the anions we find the limiting value of the radius for a particular geometry which is known as the limiting radius ratio rule. Its application is in the prediction of crystal geometry. It also gives us an idea about thermal stability of carbonates, tendency of metal ions to form hydrated salts etc.

Limiting radius ratio of cation to anion	Structure
0 - 0.155	Linear
0.155 - 0.225	Trigonal planar
0.225 - 0.414	Tetrahedral
0.414 - 0.732	Octahedral
0.414 – 0.732	Square planar
0.732 – 0.1	Body centred cubic.