
Eight resistances each of
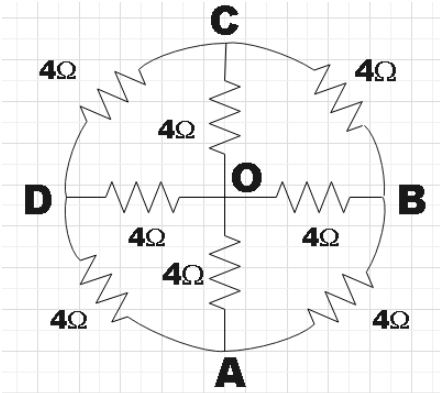
A.
B.
C.
D.

Answer
467.4k+ views
Hint: You could first find the node about which there is symmetry in the given figure. By removing the connection accordingly, you could go for finding the parallel and series connections present within the circuit. You could then reduce the complex combination into a rather simple one and thus find the answer.
Formula used:
Effective resistance:
Series,
Parallel,
Complete Step by step solution:
In the question, we are given a combination of eight resistors that are connected to each other using parallel and series connections. We are asked to find the effective resistance of the combination across the terminals A and B.
Let us proceed with the normal steps that we usually follow while finding the effective resistance. We could first simplify the given circuit. For that let us recall that when there is symmetry across a node, then we could disconnect and it will make no difference. Consider the given combination, we see that there is symmetry across the point O. We could disconnect at that node such that it will make no effect. The circuit will now look like this:
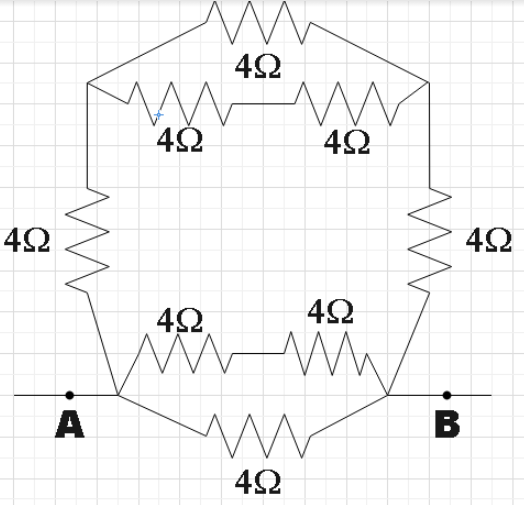
Now, we know that, the effective resistance for a series combination and parallel combination are respectively given by,
Now this circuit can be redrawn as,
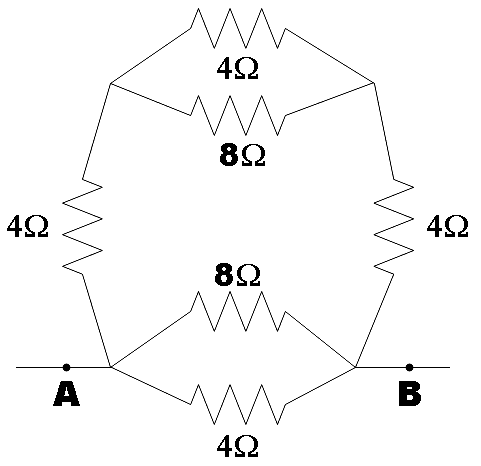
This can again be reduced as,
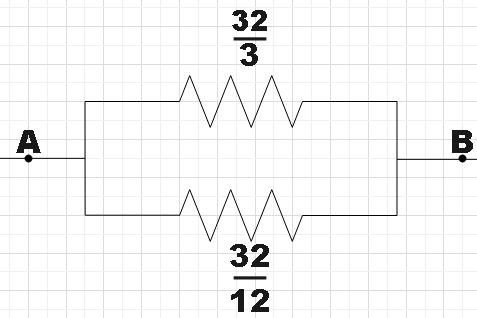
So we have reduced the complex combination step by step into a simple parallel connection and the effective resistance will now be,
Therefore, we have found the effective resistance of the circuit to be
Hence, option C is found to be the answer.
Note:
Though we haven’t mentioned finding the effective resistance at each step, we have to understand there is no complicated step involved in each reduction of the circuit. We have simply applied the above mentioned relations as per requirement. So, one shouldn’t be worried when he/she sees the complex circuit as it can easily be reduced to a very simple one.
Formula used:
Effective resistance:
Series,
Parallel,
Complete Step by step solution:
In the question, we are given a combination of eight resistors that are connected to each other using parallel and series connections. We are asked to find the effective resistance of the combination across the terminals A and B.
Let us proceed with the normal steps that we usually follow while finding the effective resistance. We could first simplify the given circuit. For that let us recall that when there is symmetry across a node, then we could disconnect and it will make no difference. Consider the given combination, we see that there is symmetry across the point O. We could disconnect at that node such that it will make no effect. The circuit will now look like this:

Now, we know that, the effective resistance for a series combination and parallel combination are respectively given by,
Now this circuit can be redrawn as,

This can again be reduced as,

So we have reduced the complex combination step by step into a simple parallel connection and the effective resistance will now be,
Therefore, we have found the effective resistance of the circuit to be
Hence, option C is found to be the answer.
Note:
Though we haven’t mentioned finding the effective resistance at each step, we have to understand there is no complicated step involved in each reduction of the circuit. We have simply applied the above mentioned relations as per requirement. So, one shouldn’t be worried when he/she sees the complex circuit as it can easily be reduced to a very simple one.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




