
Electric intensity is –
A. a scalar quantity
B. a vector quantity
C. neither scalar nor vector
D. sometimes scalar and sometimes vector
Answer
515.7k+ views
Hint: Electric field is the force experienced by a unit positive charge kept at that point. Relation between electric field intensity E, force F and q is given by
Complete step-by-step answer:
Electric field at a point is measured in terms of Electric field intensity or electric intensity E. Electric intensity in an electric field at a point is the force experienced by a unit positive charge kept at that point.
Now, we consider point charge placed at point O in air as shown in figure. A test charge
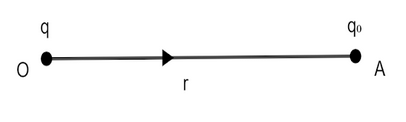
According to Coulomb’s law, the test charge
By definition, electric intensity at point B is given as,
Using equation (1) and (2) we can write,
The direction of F is along the line joining the points O and A, passing away from the charge q if q is positive and toward q, if q is negative. So, the direction is the same as force.
Hence, electric intensity is a vector quantity.
Force in vector form,
Electric intensity in vector form is,
where,
Note: Electric intensity is also known as electric field and electric field intensity. Every charged particle creates an electric field around it in which the effect of electric force is experienced by another charge. The SI unit of electric intensity is Newton per coulomb and dimension is
Complete step-by-step answer:
Electric field at a point is measured in terms of Electric field intensity or electric intensity E. Electric intensity in an electric field at a point is the force experienced by a unit positive charge kept at that point.
Now, we consider point charge placed at point O in air as shown in figure. A test charge

According to Coulomb’s law, the test charge
By definition, electric intensity at point B is given as,
Using equation (1) and (2) we can write,
The direction of F is along the line joining the points O and A, passing away from the charge q if q is positive and toward q, if q is negative. So, the direction is the same as force.
Hence, electric intensity is a vector quantity.
Force in vector form,
Electric intensity in vector form is,
where,
Note: Electric intensity is also known as electric field and electric field intensity. Every charged particle creates an electric field around it in which the effect of electric force is experienced by another charge. The SI unit of electric intensity is Newton per coulomb and dimension is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




