
What is the electric potential energy of the charge configuration as shown in the figure? Assume that
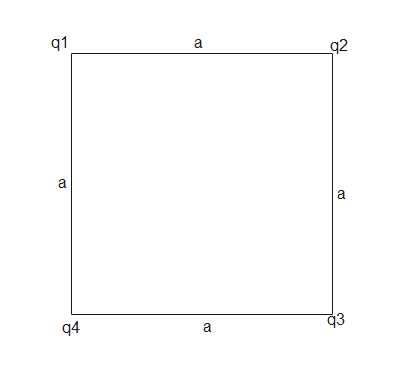

Answer
421.8k+ views
Hint: We know that the potential difference between two points in any electrical circuit is defined as the energy required to move a unit positive charge between the two points and potential energy is the energy stored by the system to do the same. Here, using the formula for the potential energy , we can find the net potential energy at the centre of the square as discussed below.
Formula used:
Complete step by step solution:
Given that,
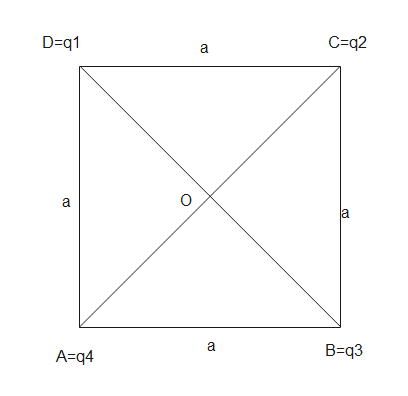
Then the potential difference at the O due to the charges will be the sum of potential energies at the centre which is given as
Substituting, for
On further simplification, we have
Since
Thus the required total potential energy at the centre of the given square with side
Additional Information:
We also know that electric current is produced due to motion of charges. The two are related by Ohm's law.
Note: If the unit positive charge is moved from a region of high potential to a region of low potential, then the energy is emitted during the process, or work is done by the system. Similarly, if the unit positive charge is moved from a region of low potential to high potential, then energy is absorbed, or work is done on the system.
Formula used:
Complete step by step solution:
Given that,

Then the potential difference at the O due to the charges will be the sum of potential energies at the centre which is given as
Substituting, for
On further simplification, we have
Since
Thus the required total potential energy at the centre of the given square with side
Additional Information:
We also know that electric current is produced due to motion of charges. The two are related by Ohm's law.
Note: If the unit positive charge is moved from a region of high potential to a region of low potential, then the energy is emitted during the process, or work is done by the system. Similarly, if the unit positive charge is moved from a region of low potential to high potential, then energy is absorbed, or work is done on the system.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




