
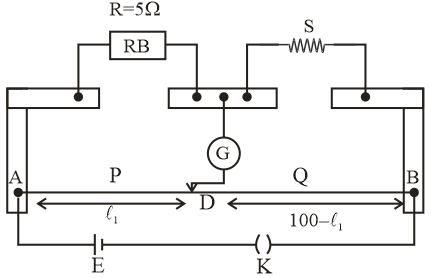
What is the end error in meter bridge ? How to overcome it ? The resistances in two arms of meter bridge are

Answer
498.6k+ views
Hint:A meter bridge end error occurs due to shifting of zero. We will write the proper definition in the solution section. Here we can understand what is the end error in simple language.See, we need to stretch the 1m wire in between two clamps so, to fix the wire we will make a loop on both the sides around the clamping screw. So a little length of wire can be more or less on both sides.And now the effective lengths of the meter bridge can be
Complete step by step answer:
End error : The shifting of zero of the scale at different points as well as the stray resistance due to non-uniformity of metal wire gives rise to end error in meter bridge.
How to overcome and error
End error can be removed by repeating the experiment by interchanging unknown and known resistance and finally taking the mean value of resistance obtained in each experiment.
Now we will solve the second part of the given question.
It is given that resistance of two arms are
By wheatstone bridge principle
Where
So,
After showing resistance S by equal resistance, the new balancing length is
So, after shunting :
Now resistance,
Or,
It is given that now balancing length is
Using whether stone bridge principle,
Or,
Here,
So,
From equation (1) and (2)
By substituting value of
In equation (1) i.e.,
It is given that
Therefore the value of S is
Note: To get balancing length of meter bridge, deflection in galvanometer should be 0. Only then we can use the formula of wheatstone bridge.

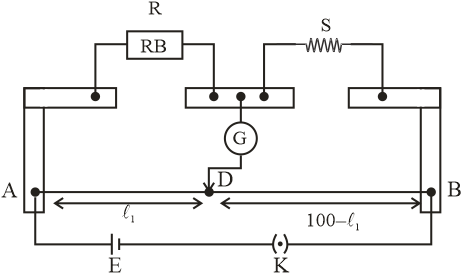
Complete step by step answer:
End error : The shifting of zero of the scale at different points as well as the stray resistance due to non-uniformity of metal wire gives rise to end error in meter bridge.
How to overcome and error
End error can be removed by repeating the experiment by interchanging unknown and known resistance and finally taking the mean value of resistance obtained in each experiment.
Now we will solve the second part of the given question.
It is given that resistance of two arms are
By wheatstone bridge principle
Where
So,
After showing resistance S by equal resistance, the new balancing length is
So, after shunting :
Now resistance,
Or,
It is given that now balancing length is
Using whether stone bridge principle,
Or,
Here,
So,
From equation (1) and (2)
By substituting value of
In equation (1) i.e.,
It is given that
Therefore the value of S is
Note: To get balancing length of meter bridge, deflection in galvanometer should be 0. Only then we can use the formula of wheatstone bridge.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




