
What is equivalent resistance? Draw the expression for effective resistance of two resistors connected in parallel.
Answer
509.1k+ views
Hint: We calculate equivalent resistance when there are combinations of resistances in a circuit. Using the concept of total voltage and total current we can calculate equivalent resistance in a circuit with the help of Ohm’s law.
Formula used:
For series combination,
For parallel combination,
Complete step by step answer:
When the circuit is designed in different combinations, series or parallel, we have to calculate the aggregate resistance, this value is known as equivalent resistance. Electrical resistance represents the amount of energy required to move charge or current in an electrical circuit. The equivalent resistance in a circuit or network is the value of that single resistor that can replace the whole network in such a way that for a certain value of applied voltage, we will get the same value of current as we would get in the original network.
Two ways in which resistances can be connected in a circuit are:
Series combination – Resistances are connected end to end along the same line. The value of current through each resistance remains the same. Value of voltage drop is different for each resistance.

Equivalent resistance formula for resistances connected in series:
Parallel combination – In this combination ends of resistances are connected at the same point. Current through each resistance will be different. Voltage across all the resistances will remain the same.
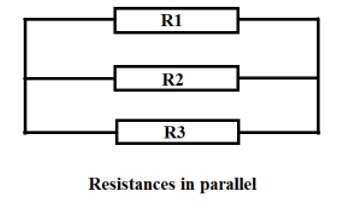
Equivalent resistance formula for resistances connected in parallel:
Let us consider two resistors
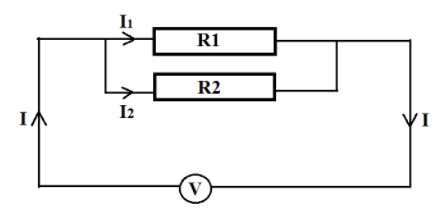
Current passing through
Total current in the circuit
Put,
Hence, the effective resistance of two resistors connected in parallel is given by:
Note: Students should keep in mind the concept of series and parallel combination; how resistances are joined in each case. Also remember, how voltage and current varies in each case. In parallel combination, voltage across each resistance remains the same while current gets divided. In series combination, current through each resistance remains the same while the voltage gets divided.
Formula used:
For series combination,
For parallel combination,
Complete step by step answer:
When the circuit is designed in different combinations, series or parallel, we have to calculate the aggregate resistance, this value is known as equivalent resistance. Electrical resistance represents the amount of energy required to move charge or current in an electrical circuit. The equivalent resistance in a circuit or network is the value of that single resistor that can replace the whole network in such a way that for a certain value of applied voltage, we will get the same value of current as we would get in the original network.
Two ways in which resistances can be connected in a circuit are:
Series combination – Resistances are connected end to end along the same line. The value of current through each resistance remains the same. Value of voltage drop is different for each resistance.

Equivalent resistance formula for resistances connected in series:
Parallel combination – In this combination ends of resistances are connected at the same point. Current through each resistance will be different. Voltage across all the resistances will remain the same.

Equivalent resistance formula for resistances connected in parallel:
Let us consider two resistors

Current passing through
Total current in the circuit
Put,
Hence, the effective resistance of two resistors connected in parallel is given by:
Note: Students should keep in mind the concept of series and parallel combination; how resistances are joined in each case. Also remember, how voltage and current varies in each case. In parallel combination, voltage across each resistance remains the same while current gets divided. In series combination, current through each resistance remains the same while the voltage gets divided.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




