Question

How do I estimate the area under the graph of \[f\left( x \right){\text{ }} = \;3cos(x)\] from \[x = 0\;\] to \[x = \dfrac{\pi }{2}\] using left and right endpoint methods?

Answer 1

Hint: In this question we have found the area of the given trigonometric form. Next, we use left and right endpoint methods and then simplify to arrive at our final answer. And also we are going to draw a graph in complete step by step solution.The area A of the region under the graph of f above the $x = a{\text{ to b}}$ can be found by
\[A = \mathop {\lim }\limits_{n \to \infty } \sum\limits_{i = 1}^n {f({x_i})\Delta x.} \]
Were, ${x_i} = a + i\Delta x$ and $\Delta x = \dfrac{{b - a}}{n}.$

Complete step by step solution:
You'll need a value for n to actually get an answer, but here's the method:
Given,
\[ \Rightarrow f\left( x \right){\text{ }} = \;3cos(x)\], \[x = 0\;\]and \[x = \dfrac{\pi }{2}\]
Find $\Delta x$:
$\Delta x = \dfrac{{b - a}}{n} = \dfrac{{\dfrac{\pi }{2} - 0}}{n} = \dfrac{\pi }{{2n}}$
Find all endpoints: start at a and successively add $\Delta x$ until you get to b:
\[0,\dfrac{\pi }{{2n}},\dfrac{{2\pi }}{{2n}},\dfrac{{3\pi }}{{2n}}, \cdot \cdot \cdot ,\dfrac{{(n - 1)\pi }}{{2n}},\dfrac{{n\pi }}{{2n}} = \dfrac{\pi }{2}\]
Each rectangle has areas base time height, which will be $\Delta x$ times f at an endpoint
To use left endpoints delete the last endpoint, above, because it is not a left endpoint
Rectangle 1 has area \[\Delta xf(0) = \left( {\dfrac{\pi }{{2n}}} \right)3cos(0)\]
Rectangle 2 has area \[\Delta xf\left( {\dfrac{\pi }{{2n}}} \right) = \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{\pi }{{2n}}} \right)\]
Rectangle 3 has area \[\Delta xf\left( {\dfrac{{2\pi }}{{2n}}} \right) = \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{{2\pi }}{{2n}}} \right)\]
Rectangle 4 has area \[\Delta xf\left( {\dfrac{{3\pi }}{{2n}}} \right) = \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{{3\pi }}{{2n}}} \right)\]
And so on up to
Rectangle n has area\[\Delta xf\left( {\dfrac{{\left( {n - 1} \right)\pi }}{{2n}}} \right) = \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{{\left( {n - 1} \right)\pi }}{{2n}}} \right)\]
Do the arithmetic and add the areas.
For right endpoints delete the case \[x = 0\;\]and add the case \[x = \dfrac{\pi }{2}\]
\[\left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{\pi }{{2n}}} \right) + \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{{2\pi }}{{2n}}} \right) + \left( {\dfrac{\pi }{{2n}}} \right)3cos\left( {\dfrac{{3\pi }}{{2n}}} \right) + ... + \left( {\dfrac{\pi }{2}} \right)3cos\left( {\dfrac{\pi }{{2n}}} \right)\]
You can divide the range 0 to \[\dfrac{\pi }{2}\]into a few rectangular strips and using left and right edges (endpoints) multiplied by the width of each strip calculate a minimum and a maximum area.

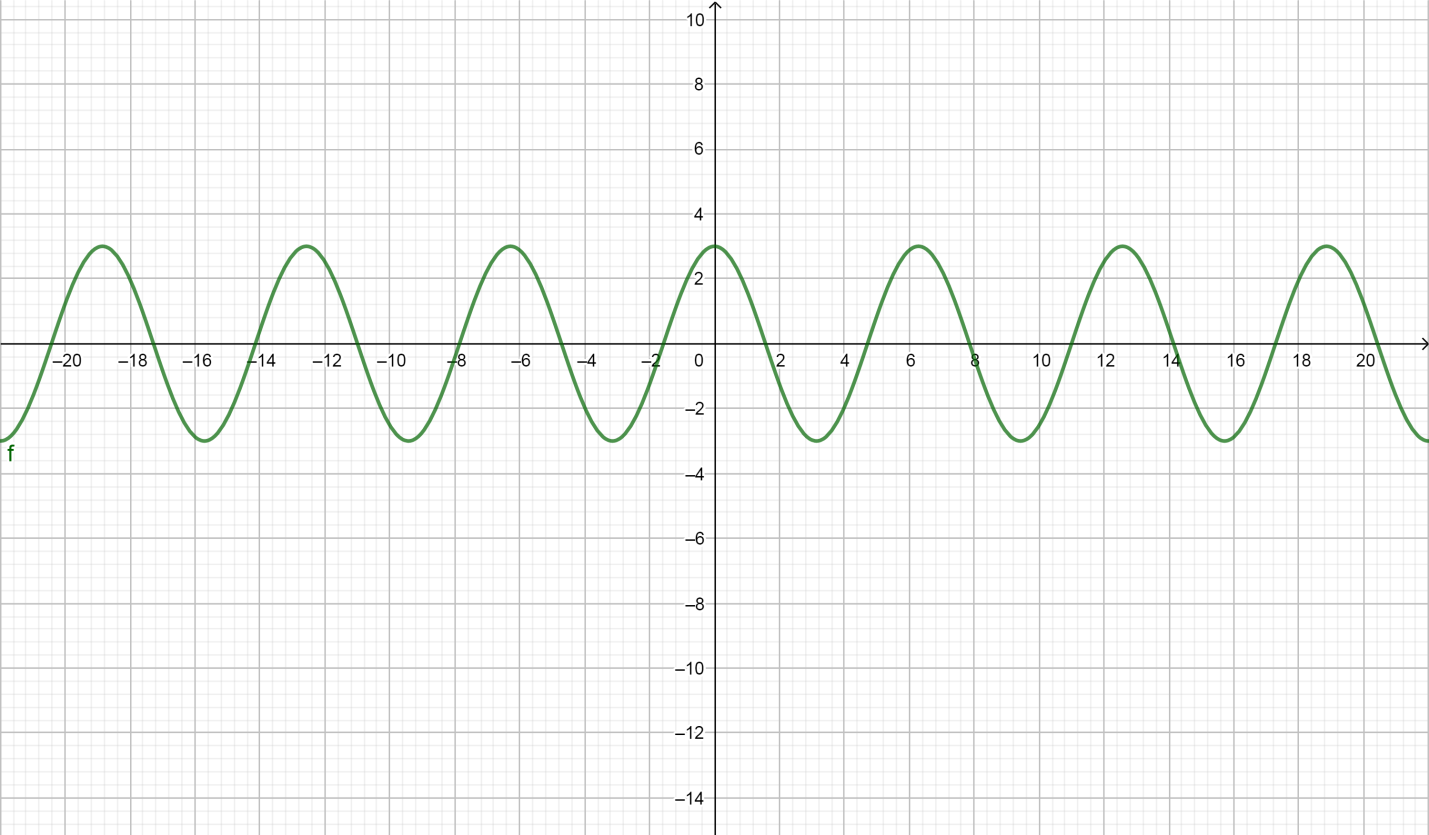
This is the required graph.

Note: We have to mind that, Rectangular integration is a numerical integration technique that approximates the integral of a function with a rectangle. It uses rectangles to approximate the area under the curve. Rectangles would be used to approximate the integral; each smaller rectangle has the width of the smaller interval.
The rectangle method (also called the midpoint rule) is the simple method in mathematics used to compute an approximation of a definite integral.