
How do you evaluate
Answer
468.6k+ views
Hint: We explain the function
Complete step-by-step solution:
The internal part
This gives in ratio
We can take the representation of a right-angle triangle with height and base ratio being 8 and the angle being
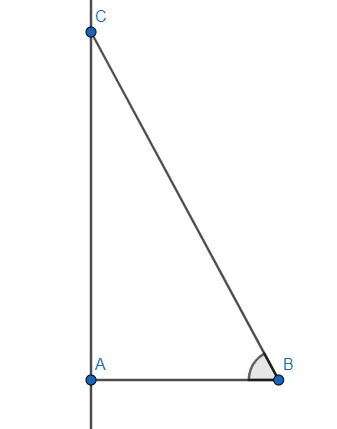
In this case we take
Now we apply the Pythagoras’ theorem to find the length of BC.
So,
We need to find
This ratio gives
Therefore,
Note: We can also apply the trigonometric image form to get the value of
It’s given that
Putting the values, we get
Complete step-by-step solution:
The internal part
This gives in ratio
We can take the representation of a right-angle triangle with height and base ratio being 8 and the angle being

In this case we take
Now we apply the Pythagoras’ theorem to find the length of BC.
So,
We need to find
This ratio gives
Therefore,
Note: We can also apply the trigonometric image form to get the value of
It’s given that
Putting the values, we get
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What are the major means of transport Explain each class 12 social science CBSE




