
What is the excess pressure inside a bubble of soap solution of radius 5.00 mm, given that the surface tension of soap solution at the temperature (20°C) is
Answer
491.1k+ views
1 likes
Hint: Before attempting the question, prior knowledge of the relation between pressure and surface tension is must according to which
Complete step-by-step solution:
According to the given question we have a soap bubble of radius 5 mm with surface tension
Since the relative density of soap solution is 1.20
So we can say that the density of soap solution is
For case 1:
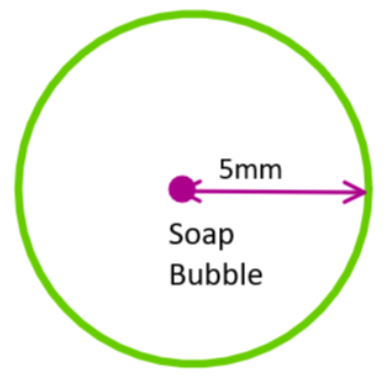
We have radius of soup bubble = 5.00 mm =
Surface tension at temp (20°C) of soup bubble is, S =
So by the relation between excess pressure inside the bubble and surface tension acting on it is given by
Since in the case of soup bubble we have 2 surfaces – inside and out therefore the relation between excess pressure inside the soap bubble and surface tension is given by
Substituting the given values in the above equation
We get
Therefore the maximum pressure experienced by the soup bubble will be 20 Pa
For case 2 we have
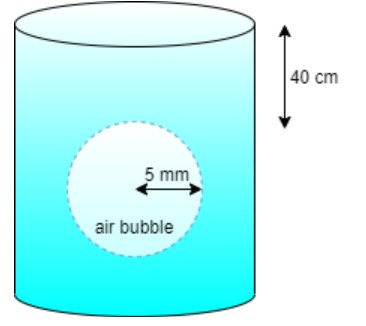
Radius of air bubble = 5.00 mm =
Depth of air bubble i.e. h = 40 cm = 0.4 m
Surface tension of air bubble is, S =
Density of soap solution =
1 atmospheric pressure is given =
Given acceleration due to gravity = 9.8 m/
By the relation between excess pressure inside air bubble and surface tension we can say that
Substituting the given values in the above equation
We get,
So the excess pressure inside the bubble made of air is equal to 10 Pa
Since we know that in this case inside the solution of soup the bubble will experience pressure due to soap solution, atmospheric pressure and pressure inside the air bubble
Therefore total pressure inside the bubble made of air is given by
Taking equation
Substituting the given values in the equation 1
We get
Hence the total pressure inside the bubble will be
Note: The term surface tension that we came across in the above solution can be explained as the characteristics of a liquid which oppose the effect of force that is applied externally, the cohesive nature of liquid is the reason behind the resisting nature of liquid towards the external forces applied. For example, when a bottle is kept on the surface of liquid it keeps floating on the surface this happens because of the cohesive nature of the liquid.
Complete step-by-step solution:
According to the given question we have a soap bubble of radius 5 mm with surface tension
Since the relative density of soap solution is 1.20
So we can say that the density of soap solution is
For case 1:

We have radius of soup bubble = 5.00 mm =
Surface tension at temp (20°C) of soup bubble is, S =
So by the relation between excess pressure inside the bubble and surface tension acting on it is given by
Since in the case of soup bubble we have 2 surfaces – inside and out therefore the relation between excess pressure inside the soap bubble and surface tension is given by
Substituting the given values in the above equation
We get
Therefore the maximum pressure experienced by the soup bubble will be 20 Pa
For case 2 we have

Radius of air bubble = 5.00 mm =
Depth of air bubble i.e. h = 40 cm = 0.4 m
Surface tension of air bubble is, S =
Density of soap solution =
1 atmospheric pressure is given =
Given acceleration due to gravity = 9.8 m/
By the relation between excess pressure inside air bubble and surface tension we can say that
Substituting the given values in the above equation
We get,
So the excess pressure inside the bubble made of air is equal to 10 Pa
Since we know that in this case inside the solution of soup the bubble will experience pressure due to soap solution, atmospheric pressure and pressure inside the air bubble
Therefore total pressure inside the bubble made of air is given by
Taking equation
Substituting the given values in the equation 1
We get
Hence the total pressure inside the bubble will be
Note: The term surface tension that we came across in the above solution can be explained as the characteristics of a liquid which oppose the effect of force that is applied externally, the cohesive nature of liquid is the reason behind the resisting nature of liquid towards the external forces applied. For example, when a bottle is kept on the surface of liquid it keeps floating on the surface this happens because of the cohesive nature of the liquid.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

In northern hemisphere 21st March is called as A Vernal class 11 social science CBSE




