
Figure shows a conducting loop
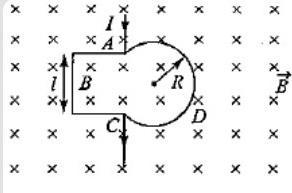
A.
B.
C.
D.

Answer
505.2k+ views
Hint: Since, the current through the loop
Useful formula:
Relation between force, current and magnetic field will be given by,
Where,
Complete Step by step solution:
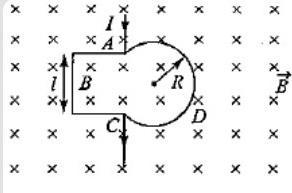
Assume that,
The current through the loop
The current through the loop
The length where the current flow in loop
The length where the current flow in loop
The force generated on the loop
Where,
The force generated on the loop
Where,
Hence, the total force on loop
Substitute the values of (1) and (2) equation (1),
Since, the current travels the same length in the loops
Hence,
The total current,
Thus,
Hence, the option (B) is correct.
Note: In the loop
Useful formula:
Relation between force, current and magnetic field will be given by,
Where,
Complete Step by step solution:

Assume that,
The current through the loop
The current through the loop
The length where the current flow in loop
The length where the current flow in loop
The force generated on the loop
Where,
The force generated on the loop
Where,
Hence, the total force on loop
Substitute the values of (1) and (2) equation (1),
Since, the current travels the same length in the loops
Hence,
The total current,
Thus,
Hence, the option (B) is correct.
Note: In the loop
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




