
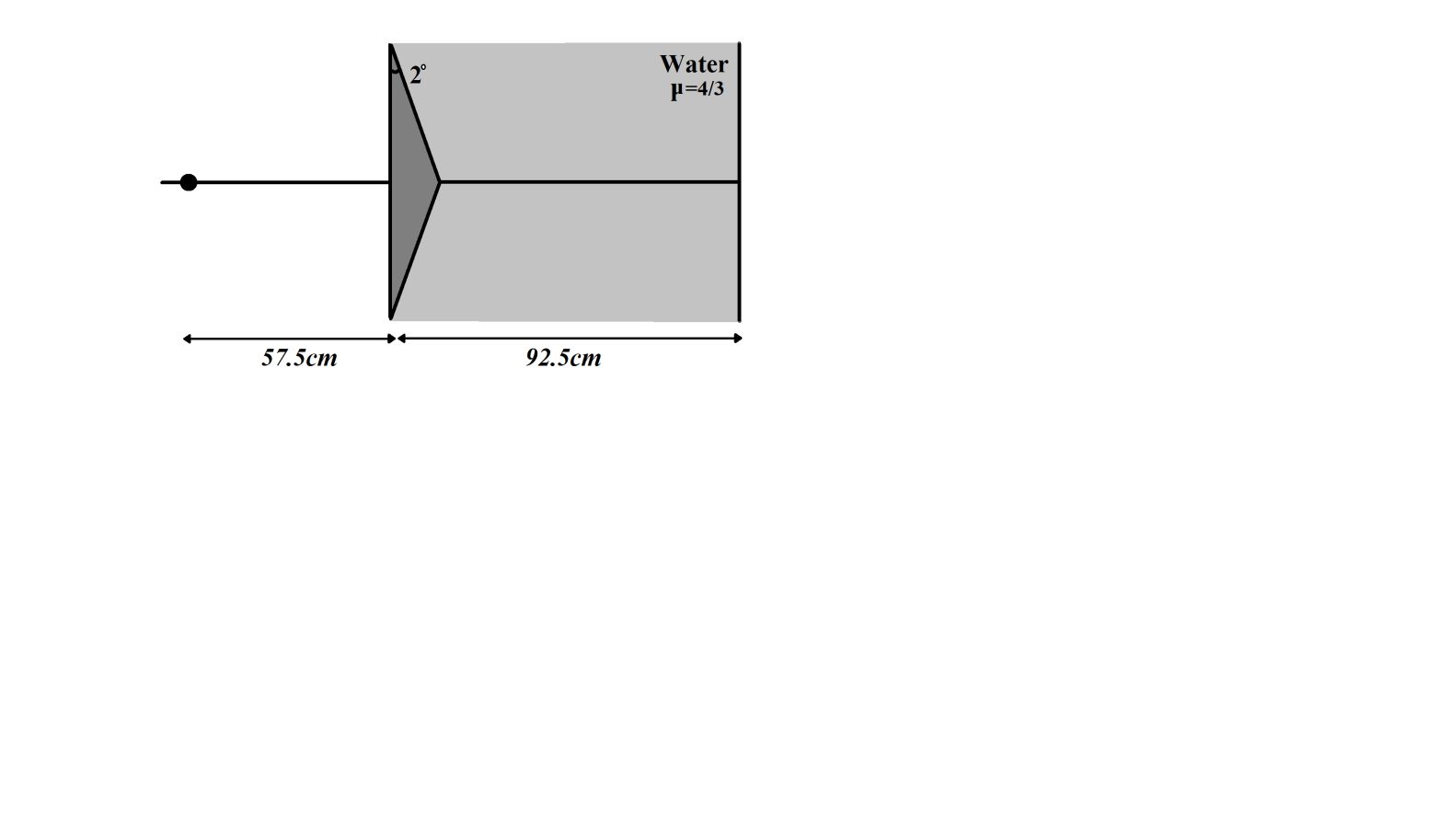
Figure shows a modified Fresnel biprism experiment with monochromatic source of wavelength

Answer
504.9k+ views
Hint: When a monochromatic light source is kept in front of biprism, two coherent virtual sources are produced. Fringe width is described as the distance between two consecutive dark fringes or two consecutive bright fringes obtained on the screen. We will use the expression for fringe width relating the wavelength of incident light, distance between the coherent sources and distance between the source and the screen.
Formula used:
Fringe width,
Angle of deviation of prism with water on one side is,
Distance between the sources,
Complete step by step solution:
Fresnel biprism is an optical device for producing interference of light waves. It can be formed by joining base to base two thin prisms of very small refracting angles.
When a monochromatic light source is kept in front of biprism, two coherent virtual sources are produced. Interference fringes are obtained on the screen placed behind the prism. Interference pattern is observed on a limited region of the screen.
Fringe width is described as the distance between two consecutive dark fringes or two consecutive bright fringes obtained on the screen.
Fringe width is given as,
Where,
We are given a Fresnel biprism having refracting angle
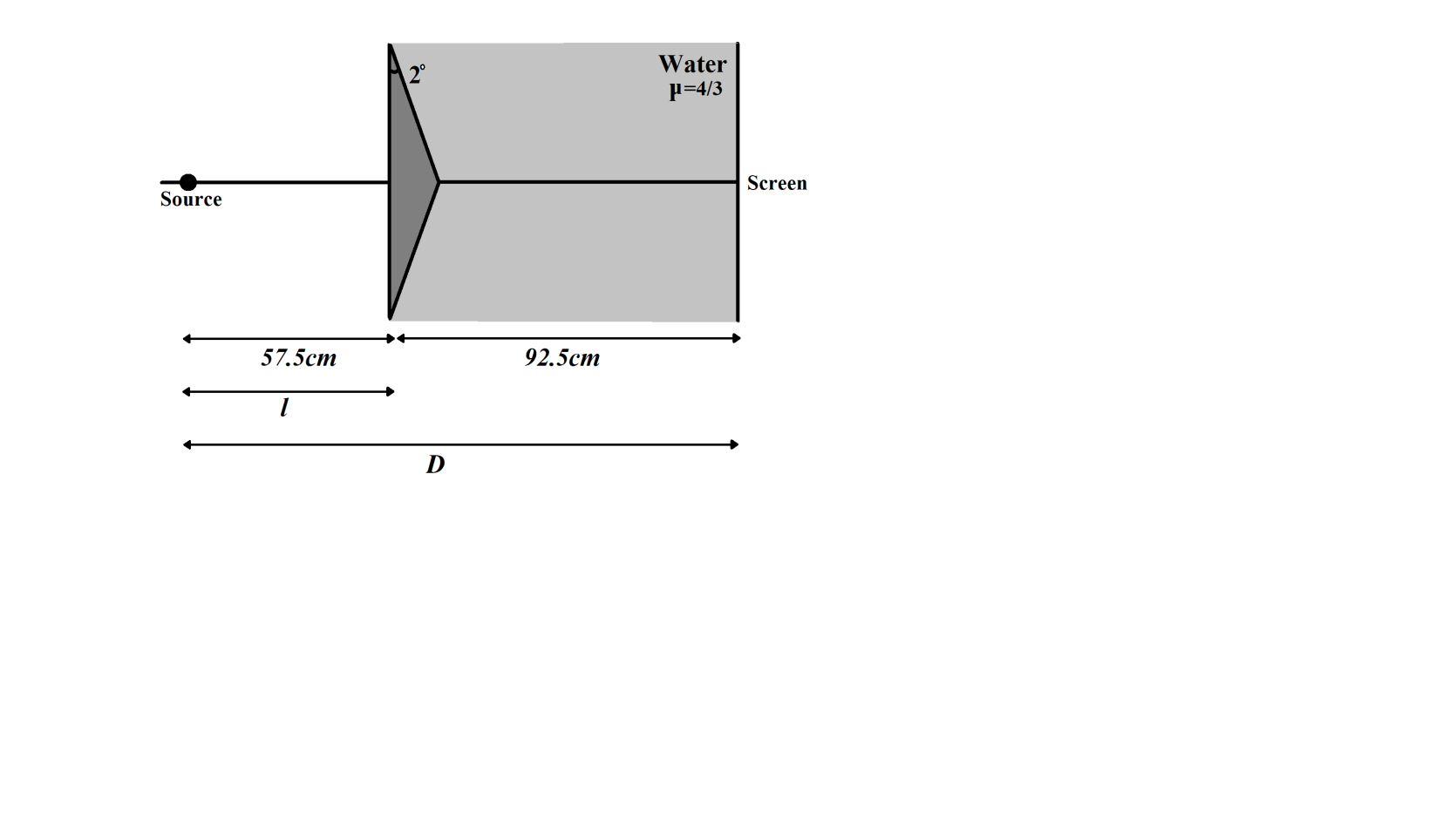
Refractive index of glass,
Angle of deviation of prism with water on one side is,
Where,
Putting values of
Now,
Where,
Putting values of
Now,
Fringe width is given as,
Where,
Putting values,
The fringe width of the given experimental set up is
Hence, the correct option is B.
Note:
Fringe width is the distance between two consecutive dark fringes or two consecutive bright fringes. In case of a hollow prism or fluid prism, relative refractive index has to be considered, in place of absolute refractive index, while calculating angle of deviation of the prism.
Formula used:
Fringe width,
Angle of deviation of prism with water on one side is,
Distance between the sources,
Complete step by step solution:
Fresnel biprism is an optical device for producing interference of light waves. It can be formed by joining base to base two thin prisms of very small refracting angles.
When a monochromatic light source is kept in front of biprism, two coherent virtual sources are produced. Interference fringes are obtained on the screen placed behind the prism. Interference pattern is observed on a limited region of the screen.
Fringe width is described as the distance between two consecutive dark fringes or two consecutive bright fringes obtained on the screen.
Fringe width is given as,
Where,
We are given a Fresnel biprism having refracting angle

Refractive index of glass,
Angle of deviation of prism with water on one side is,
Where,
Putting values of
Now,
Where,
Putting values of
Now,
Fringe width is given as,
Where,
Putting values,
The fringe width of the given experimental set up is
Hence, the correct option is B.
Note:
Fringe width is the distance between two consecutive dark fringes or two consecutive bright fringes. In case of a hollow prism or fluid prism, relative refractive index has to be considered, in place of absolute refractive index, while calculating angle of deviation of the prism.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




