
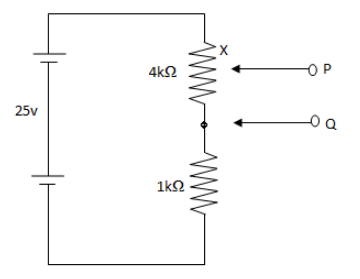
Figure shows a potential divider circuit, which by adjustment of the position of the contact X, can be used to provide a variable potential difference between the terminals P and Q. What are the limits of this potential difference?
A.
B.
C.
D.

Answer
469.5k+ views
Hint: We are asked to find the lower limit and upper limit of potential difference between the terminals P and Q. To find the lower limit move the terminal P to the lower end of resistor
Complete step by step answer:
We are given a circuit and asked to find the range of potential difference between the terminals P and Q.Let us first find the lower limit of the potential difference between the terminals P and Q.We can find the lower limit of potential difference by moving the terminal P to the lower end of
Now, we find the upper limit of potential difference between the terminals P and Q. In this case we move the terminal P to the upper end of
We redraw the circuit.
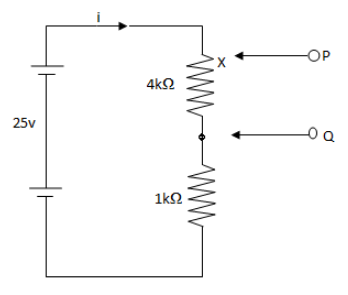
Here, the equivalent resistance will be,
Current through a circuit is,
Here, voltage is
Putting the values of voltage and equivalent resistance in equation (i) we get,
Now, voltage across the resistor
Putting the value of
Therefore, the upper limit of potential difference between the terminals P and Q is
Hence, the correct answer is option C.
Note:Using a potential divider circuit, we can get different voltages from a common supply voltage. One example of a potential divider is potentiometer. Potential divider circuits can be used for controlling audio volume, for controlling temperature in the freezer.
Complete step by step answer:
We are given a circuit and asked to find the range of potential difference between the terminals P and Q.Let us first find the lower limit of the potential difference between the terminals P and Q.We can find the lower limit of potential difference by moving the terminal P to the lower end of
Now, we find the upper limit of potential difference between the terminals P and Q. In this case we move the terminal P to the upper end of
We redraw the circuit.

Here, the equivalent resistance will be,
Current through a circuit is,
Here, voltage is
Putting the values of voltage and equivalent resistance in equation (i) we get,
Now, voltage across the resistor
Putting the value of
Therefore, the upper limit of potential difference between the terminals P and Q is
Hence, the correct answer is option C.
Note:Using a potential divider circuit, we can get different voltages from a common supply voltage. One example of a potential divider is potentiometer. Potential divider circuits can be used for controlling audio volume, for controlling temperature in the freezer.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




