
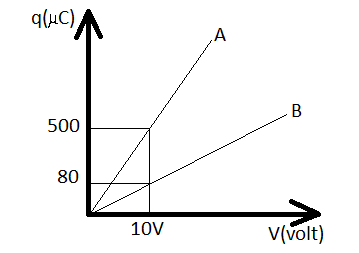
Figure shows charge (q) versus voltage (V) graph from series and parallel combination of two given capacitors. Determine the capacitances.
(A) $50\mu F$ and $30/muF$
(B) $20\mu F$ and $30/muF$
(C) $60\mu F$ and $40/muF$
(D) $40\mu F$ and $10/muF$
Answer
123k+ views
Hint In the figure a charge (q) versus voltage (V) graph from series and parallel combination of two capacitors is given. In order to find out the value of the two capacitors, we must use the formula $q = cV$, where q is the charge of the capacitor, V potential difference across two plates and c is the capacitance.
Complete step by step answer
We know that the charge q stored on either plate of a capacitor is directly proportional to the potential difference V across two plates, $q = cV$ where q is charge of the capacitor, V potential difference across two plates and c is the capacitance.
Here the slope of the graph will give capacitance. Now we have to determine capacitance for series and parallel combination.
In series combination,
$\dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = \dfrac{q}{V}$
$ \Rightarrow \dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = \dfrac{{80}}{{10}}$
$ \Rightarrow \dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = 8 \times {10^{ - 6}}F$……. (i)
In parallel combination,
${C_1} + {C_2} = \dfrac{q}{V}$
$ = \dfrac{{500}}{{10}}$
$ = 50 \times {10^{ - 6}}F$
Putting the value of ${C_1} + {C_2}$ in equation (i) we get ${C_1}{C_2} = 400 \times {10^{ - 6}}F$
Therefore, capacitance in parallel should be 50/muF & capacitance in series must be 8μF.
It is only possible when ${C_1} = 10\mu F$ and ${C_2} = 40\mu F$
hence option D is correct.
Additional Information $q = cV$, here c is a constant of proportionality called the capacitance of the capacitor. The SI unit of capacitance is farad. 1 Farad = 1 coulomb/volt. The capacitance of a capacitor depends upon the area of the plates, the distance between the plates and the medium between them.
Note Whenever these types of questions appear, first thoroughly examine the graph. From the graph it can be easily determined that the slope of the graph gives capacitance as X and Y-axis represent voltage and charge respectively. Then determine the capacitance for series and parallel combination separately.
Complete step by step answer
We know that the charge q stored on either plate of a capacitor is directly proportional to the potential difference V across two plates, $q = cV$ where q is charge of the capacitor, V potential difference across two plates and c is the capacitance.
Here the slope of the graph will give capacitance. Now we have to determine capacitance for series and parallel combination.
In series combination,
$\dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = \dfrac{q}{V}$
$ \Rightarrow \dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = \dfrac{{80}}{{10}}$
$ \Rightarrow \dfrac{{{C_1}{C_2}}}{{{C_1} + {C_2}}} = 8 \times {10^{ - 6}}F$……. (i)
In parallel combination,
${C_1} + {C_2} = \dfrac{q}{V}$
$ = \dfrac{{500}}{{10}}$
$ = 50 \times {10^{ - 6}}F$
Putting the value of ${C_1} + {C_2}$ in equation (i) we get ${C_1}{C_2} = 400 \times {10^{ - 6}}F$
Therefore, capacitance in parallel should be 50/muF & capacitance in series must be 8μF.
It is only possible when ${C_1} = 10\mu F$ and ${C_2} = 40\mu F$
hence option D is correct.
Additional Information $q = cV$, here c is a constant of proportionality called the capacitance of the capacitor. The SI unit of capacitance is farad. 1 Farad = 1 coulomb/volt. The capacitance of a capacitor depends upon the area of the plates, the distance between the plates and the medium between them.
Note Whenever these types of questions appear, first thoroughly examine the graph. From the graph it can be easily determined that the slope of the graph gives capacitance as X and Y-axis represent voltage and charge respectively. Then determine the capacitance for series and parallel combination separately.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility & More

JEE Main Login 2045: Step-by-Step Instructions and Details

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Charging and Discharging of Capacitor

Physics Average Value and RMS Value JEE Main 2025

Degree of Dissociation and Its Formula With Solved Example for JEE




