
Find out the altitude of a cylinder of the greatest possible volume which can be inscribed in a sphere of radius
Answer
487.2k+ views
Hint: We first find the relation between the diagonal length of the cylinder to the radius of the circle. This equation will help to build the function of the volume of the cylinder to find its maximum value possible. We then apply the theorem of maxima and minima to find the possible value of the altitude. We have to apply the differentiation in that case.
Complete step-by-step solution:
We know the volume of a cylinder is
Now we need to fit a cylinder of the greatest possible volume in a sphere of radius
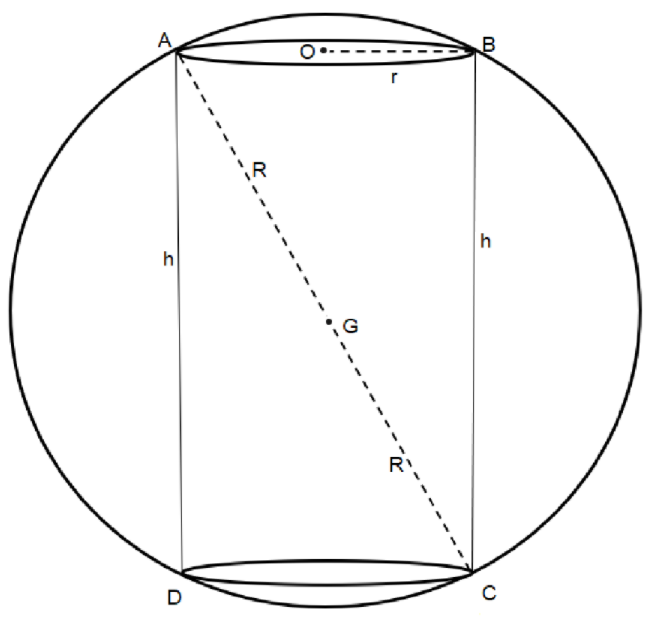
Putting the value, we get
Let’s take the volume of a cylinder as a function
We replace value of r from equation (i) into equation (ii) to get
Now we apply the theorem of maxima and minima as we need the greatest possible volume v.
So, we differentiae v with respect to h to get
We take
We solve it to get the value of h as
Now to find the value of
We put value of h in
For h = 6,
For h = -6,
So, we take the value of “h” as 6 which is the altitude of the cylinder.
Note: We need to remember the relation of the cylinder and the circle is created from the right-angle triangle. Without that relation, we can’t get the function of volume. We also have to find the value of
Complete step-by-step solution:
We know the volume of a cylinder is
Now we need to fit a cylinder of the greatest possible volume in a sphere of radius

Putting the value, we get
Let’s take the volume of a cylinder as a function
We replace value of r from equation (i) into equation (ii) to get
Now we apply the theorem of maxima and minima as we need the greatest possible volume v.
So, we differentiae v with respect to h to get
We take
We solve it to get the value of h as
Now to find the value of
We put value of h in
For h = 6,
For h = -6,
So, we take the value of “h” as 6 which is the altitude of the cylinder.
Note: We need to remember the relation of the cylinder and the circle is created from the right-angle triangle. Without that relation, we can’t get the function of volume. We also have to find the value of
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




