
Find out the centre of mass of an isosceles triangle of base length a and altitude b. Assume that the mass of the triangle is uniformly distributed over its area.
A.
B.
C.
D.
Answer
505.8k+ views
Hint: In such types of questions of variable or uniform distribution of mass we use the method integration. Doing this will solve your problem and will give you the right answer.
Formula used:
Complete step-by-step solution -
The moment of inertia is defined as the ratio of the net angular momentum of the system to its angular velocity around the main axis, that is. Unless the angular momentum of the system is unchanged, the angular velocity will increase as the moment of inertia decreases.
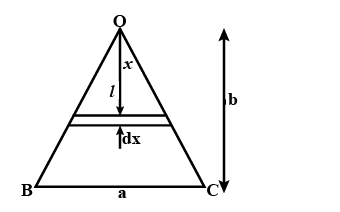
Given, base length =a
Altitude = b
To locate the center of mass of the triangle, we take a strip of width dx at a distance x from the vertex of the triangle. Length of this strip can be evaluated by similar triangles as
Mass of the strip is
Distance of center of mass from the vertex of the triangle is
Hence, the correct answer is
Note: The center of mass of a distribution of mass in space (sometimes referred to as the balance point) is the unique point where the weighted relative position of the distributed mass sums to zero. Centre of mass of a figure in X- coordinate and Y- coordinate is
Formula used:
Complete step-by-step solution -

The moment of inertia is defined as the ratio of the net angular momentum of the system to its angular velocity around the main axis, that is. Unless the angular momentum of the system is unchanged, the angular velocity will increase as the moment of inertia decreases.
Given, base length =a
Altitude = b
To locate the center of mass of the triangle, we take a strip of width dx at a distance x from the vertex of the triangle. Length of this strip can be evaluated by similar triangles as
Mass of the strip is
Distance of center of mass from the vertex of the triangle is
Hence, the correct answer is
Note: The center of mass of a distribution of mass in space (sometimes referred to as the balance point) is the unique point where the weighted relative position of the distributed mass sums to zero. Centre of mass of a figure in X- coordinate and Y- coordinate is
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




